Analyzing the Concavity of a Family of Parabolas

To examine the concavity of a family of parabolas as the parameter $\lambda$ changes, I need to look at how the coefficient of the $x^2$ term in the general equation of the family varies.

$$ Ax^2 + Bx + Cy + D = 0 $$

Where the coefficients are:

$$ A = a_1 + \lambda a_2 $$

$$ B = b_1 + \lambda b_2 $$

$$ C = 1 + \lambda $$

$$ D = c_1 + \lambda c_2 $$

The concavity of the parabola is determined by the sign of the coefficient of the $x^2$ term, which is $ A $, and the sign of the variable $ y $.

For a parabola parallel to the y-axis, if $C > 0$ then:

If $A < 0$, the parabola opens upwards.
If $A > 0$, the parabola opens downwards.

Conversely, if $C < 0$ then:

If $A < 0$, the parabola opens downwards.
If $A > 0$, the parabola opens upwards.

Regardless of the sign of $C$, if $A = 0$, the equation represents a line, not a parabola.

Note: The equation $ Ax^2 + Bx + Cy + D = 0 $ can be rearranged to $ Cy = -Ax^2 - Bx - D $. Therefore, considering for simplicity the case where $C > 0$, if $A < 0$, the coefficient $-Ax^2$ becomes positive, and the curve opens upwards (concave downwards). Conversely, when $A > 0$, it opens downwards (concave upwards).

Once the critical values of $\lambda$ are found where $ A = 0 $ and the parabola transitions from opening upwards to opening downwards (or vice versa), I use the critical value found to study the sign and determine the intervals of $\lambda$:

A Practical Example

I need to study how the concavity changes as $\lambda$ varies in the family of parabolas given by:

$$ (y - x^2 + 3x - 2) + \lambda (y + x^2 - 5x + 4) = 0 $$

I start by rewriting the equation in a simpler form. Expanding and simplifying the equation:

$$ y - x^2 + 3x - 2 + \lambda y + \lambda x^2 - 5\lambda x + 4\lambda = 0 $$

$$ (1 + \lambda)y + (\lambda-1)x^2 + (3 - 5\lambda)x + (-2 + 4\lambda) = 0. $$

This is the general equation for the family of parabolas.

For simplicity, I can rewrite it in this form:

$$ Ax^2 + Bx + Cy + D = 0 $$

Where:

$$ A = \lambda -1 $$

$$ B = 3 - 5\lambda $$

$$ C = 1 + \lambda $$

$$ D = -2 + 4\lambda $$

The concavity of a parabola depends on the sign of the coefficient of the $x^2$ term, which is $ A $:

$$ A = \lambda -1 $$

However, I also need to consider the sign of $C$ because it affects the sign of the variable $ y $:

$$ C = 1 + \lambda > 0 $$

$$ \lambda > -1 $$

Thus, the coefficient $C$ is positive in the interval (-1, ∞) and negative in the interval (-∞, -1) of the parameter $ \lambda $.

Now, let's study the sign of the term $ A $:

$$ A = \lambda -1 > 0 $$

$$ \lambda > 1 $$

Therefore, the coefficient $A$ is positive in the interval (1, ∞) and negative in the interval (-∞, 1) of the parameter $ \lambda $.

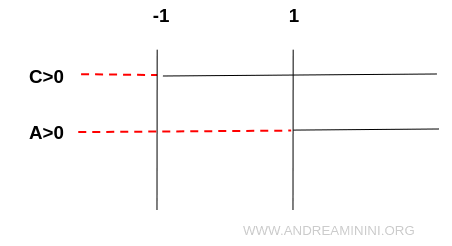
The signs of the coefficients A and C

In the interval $\lambda \in (-1, ∞)$ where the coefficient $C$ is positive:

The parabola opens downwards in the interval (1, ∞) because $ A > 0 $
The parabola opens upwards in the interval (-1, 1) because $ A < 0 $

In the interval $\lambda \in (-∞, -1)$ where the coefficient $C$ is negative:

The parabola opens downwards in the interval (-∞, 1) because $ A < 0 $

To summarize:

For $\lambda > 1$, the parabola opens downwards (concave upwards).
For $-1 < \lambda < 1$, the parabola opens upwards (concave downwards).
For $\lambda < -1$, the parabola opens downwards (concave downwards) because the $ y $ term becomes negative, affecting the overall shape of the parabola.

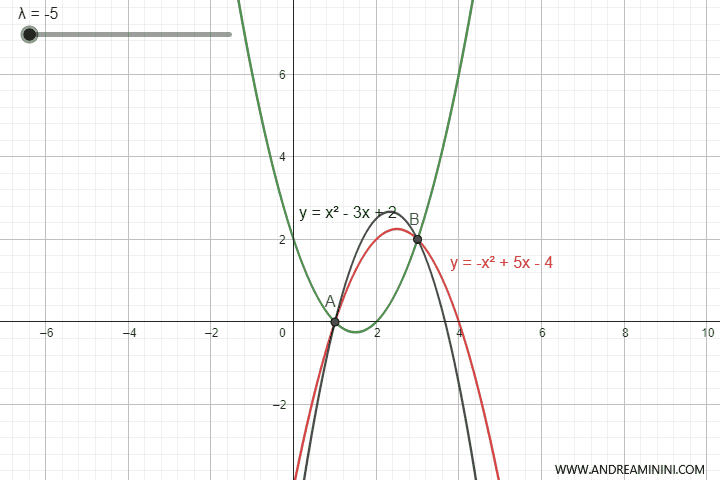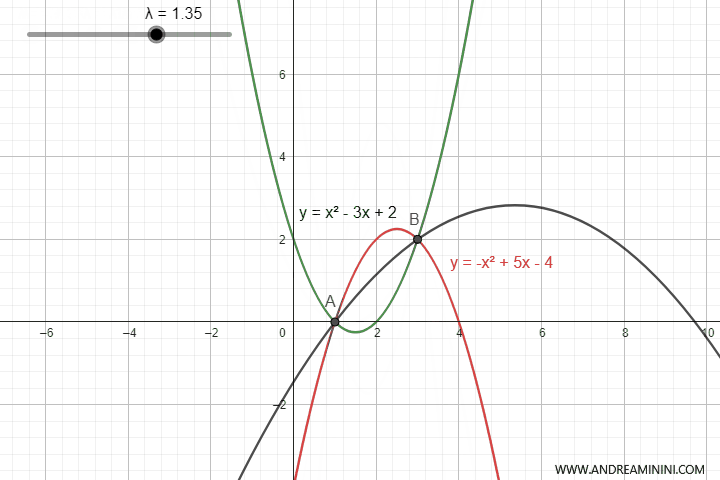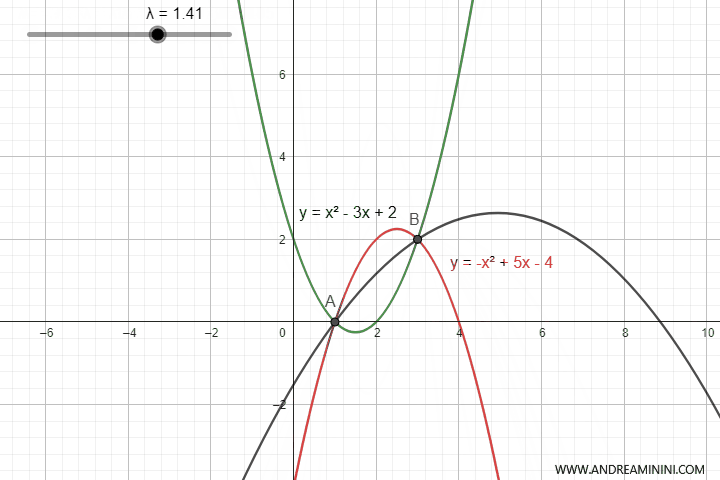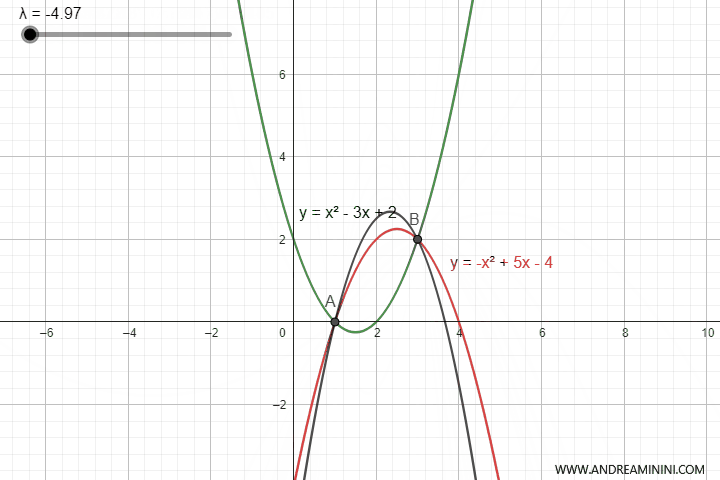
The family of parabolas

In this example, at the critical points $\lambda = 1$ and $\lambda = -1$ where the parabola transitions from concave to convex (or vice versa), the parabola degenerates into a line.

At $\lambda = 1$, the degenerate parabola passes through both base points A and B of the family, while at $\lambda = -1$, the degenerate parabola appears as a pair of lines parallel to the axis of symmetry.

Degenerate parabolas

Note: In a family of parabolas, as the parameter $\lambda$ changes, both the sign of the coefficient $A$ and the coefficient $C$ change. Both of these terms affect the opening of the parabola in the graph.

And so on.