Uncertainty and Absolute Error

The uncertainty is the interval within which an approximated value is expected to fall, accounting for both under- and over-estimations. This range signifies the absolute error or the uncertainty linked to the measurement.

When working with a real number, like pi \( r = 3.1415\ldots \), and rounding it both down and up, we create an interval that encompasses the approximate value.

For example, if the approximation is to four decimal places:

• The lower-bound approximation is \( 3.1415 \)
• The upper-bound approximation is \( 3.1416 \)

Thus, the real number \( r \) is within the uncertainty range \([3.1415; 3.1416]\), with an approximate midpoint of \( r \approx 3.14155 \).

The midpoint is the average of the lower- and upper-bound approximations. $$ \frac{3.1415+3.1416}{2} = 3.14155 $$

The half-width of this interval is \( 0.00005 \), representing the precision or uncertainty of the approximation. This is also called the maximum error or absolute error.

A Practical Example

Imagine measuring the length of an object, like a pencil, with an instrument sensitive to \( 0.1 \, \text{cm} \)—such as a standard school ruler marked in millimeters.

The recorded length is \( 15.3 \, \text{cm} \).

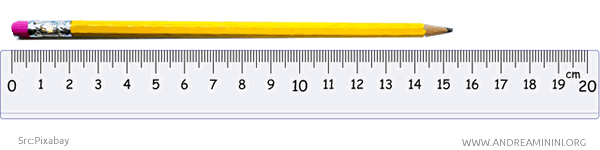
In this case, the uncertainty range is \([15.2; 15.4]\)

The estimated length is \( 15.3 \, \text{cm} \), and the absolute error is \( 0.1 \, \text{cm} \).

So, I represent this measurement as \( 15.3 \pm 0.1 \, \text{cm} \), indicating that the value measured has a maximum uncertainty of \( 0.1 \, \text{cm} \).

Example 2

Suppose I need to take a body temperature reading with a thermometer that has a precision of \( 0.1^\circ \text{C} \).

If the temperature reads \( 36.2^\circ \text{C} \), the actual value falls within the range \([36.1^\circ \text{C}; 36.3^\circ \text{C}]\).

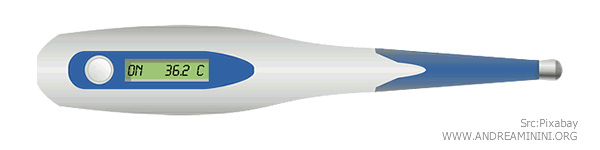
In this case, the measurement is expressed as \( 36.2 \pm 0.1^\circ \text{C} \), where \( 0.1^\circ \text{C} \) represents the maximum error associated with this reading.

And so on.