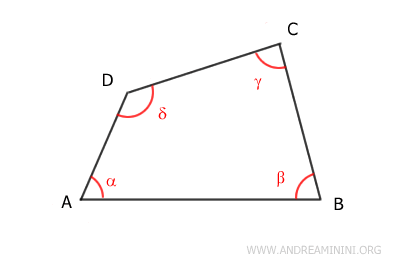
Quadrilaterals
A quadrilateral is a polygon with four sides.
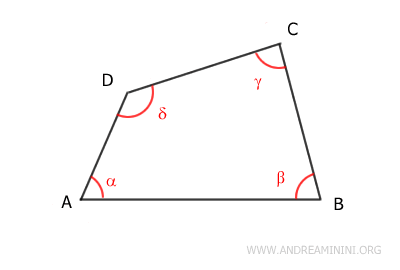
The sides of a quadrilateral can be categorized as:
- Adjacent sides
if they share a common vertex. - Opposite sides
if they do not share any vertices.
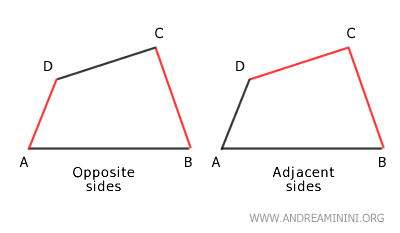
The angles in a quadrilateral can be:
- Adjacent angles
if they share a common side. - Opposite angles
if they do not share any sides.
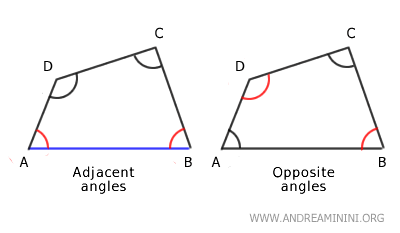
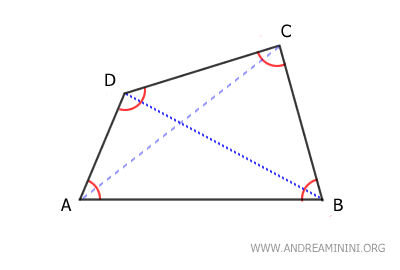
Every quadrilateral has two diagonals that connect the vertices of opposite angles.
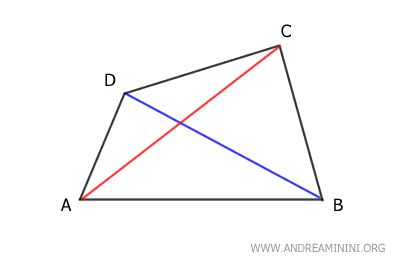
Note: A diagonal divides a quadrilateral into two triangles. Since the sum of the interior angles of a triangle is 180°, and a quadrilateral is composed of two triangles, the sum of the interior angles of a quadrilateral is 360°.
The sum of the interior angles of a quadrilateral is always 360° (a full circle).
$$ \alpha + \beta + \gamma + \delta = 360° $$
Types of Quadrilaterals
Based on their shape, quadrilaterals can be classified into:
- Convex quadrilaterals
All interior angles are less than 180° (convex), meaning that all of its diagonals lie within the quadrilateral.
Convex quadrilaterals include trapezoids, parallelograms, and convex kites.- Trapezoids
These are quadrilaterals with only one pair of parallel sides. A trapezoid can be scalene, isosceles, or right-angled.
- Parallelograms
These are quadrilaterals with opposite sides that are both parallel and equal in length.
Parallelograms include rectangles, rhombuses, and squares.- Rectangles
Parallelograms with all angles at 90°.
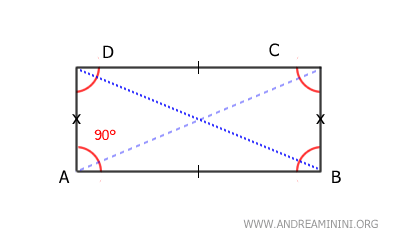
- Rhombuses
Parallelograms where all sides are of equal length and the diagonals are perpendicular.

- Squares
Parallelograms with all sides of equal length, all angles at 90°, and perpendicular diagonals. In essence, squares possess the characteristics of both rectangles and rhombuses.
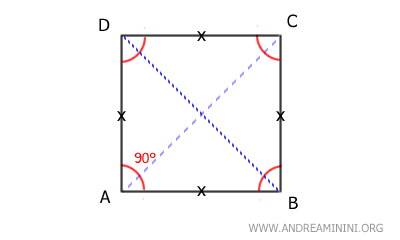
- Rectangles
- Convex kites
These are quadrilaterals with perpendicular diagonals.
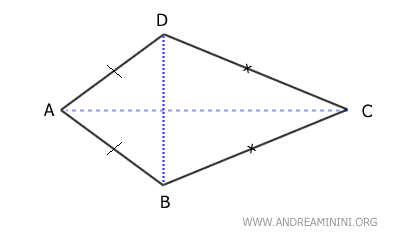
- Trapezoids
- Concave quadrilaterals
In these quadrilaterals, at least one interior angle is greater than 180° (concave), meaning that at least one diagonal lies outside the quadrilateral.
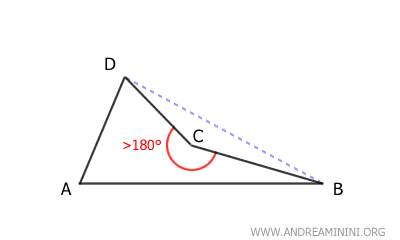
Additional Notes
Some key points about quadrilaterals:
- The perimeter of a quadrilateral is the total length of all its sides.
- Each side of a quadrilateral is shorter than the sum of the other three sides.
- Area of a quadrilateral with perpendicular diagonals
The area of a quadrilateral with perpendicular diagonals can be calculated by multiplying the lengths of the diagonals and dividing the result by two. $$ A = \frac{d_1 \cdot d_2}{2} $$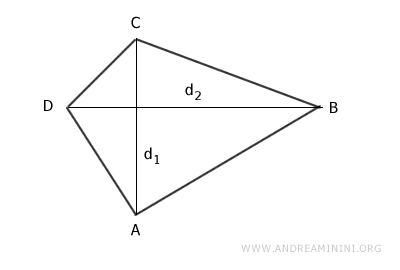
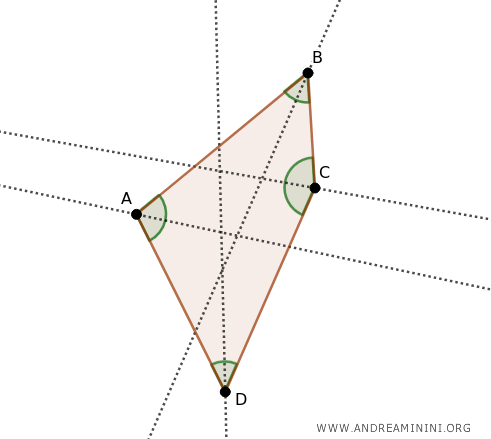
- Circumscribed Quadrilaterals
A quadrilateral is circumscribed about a circle if the angle bisectors converge at a single point, known as the "incenter." The circle, centered at this incenter (O), touches all four sides of the quadrilateral.

For instance, a rhombus and a square are examples of quadrilaterals that can be circumscribed because their angle bisectors intersect at a common point. However, not all quadrilaterals have this property. Below is an example of a quadrilateral that cannot be circumscribed.
If a quadrilateral is circumscribed by a circle, the sum of the lengths of two opposite sides is equal to the sum of the lengths of the other two sides. $$ \overline{AB} + \overline{CD} = \overline{BC} + \overline{AD} $$
And so on...
