Parallel Planes
Two planes in space are considered parallel if they meet one of the following conditions:
- They do not share any points, meaning they never intersect. These are called non-coincident parallel planes.
- They share every point, meaning they are identical. These are referred to as coincident planes.
For example, the floors of two levels in a building, assuming they are perfectly horizontal, form a pair of non-coincident parallel planes.
Distance Between Parallel Planes
The distance between two non-coincident parallel planes is the length of the perpendicular segment connecting a point on one plane to a point on the other.
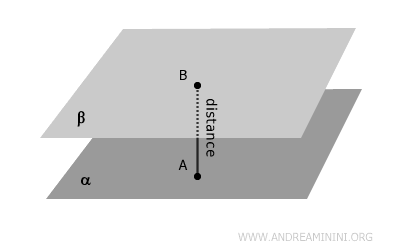
This distance is the same for all points on both planes.
How to Calculate the Distance Between Two Parallel Planes
In analytic geometry, the equations of two parallel planes can be written as:
$$ Ax + By + Cz + D_1 = 0 $$
$$ Ax + By + Cz + D_2 = 0 $$
The distance between these two planes is given by the formula:
$$ d = \frac{|D_2 - D_1|}{\sqrt{A^2 + B^2 + C^2}} $$
where \( A, B, C \) are the coefficients of the normal vector to the planes.
Note: If the distance is zero \( d=0 \), the two planes are coincident. If the distance is a positive value \( d>0 \), the planes are distinct but parallel.
Example
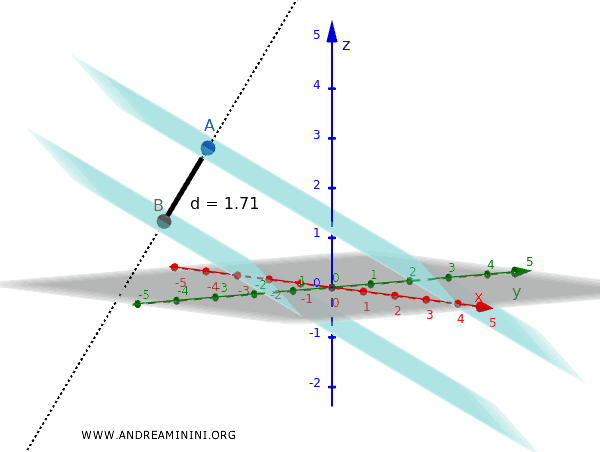
Consider two parallel planes with the following equations:
$$ 2x + 3y + 6z + 4 = 0 $$
$$ 2x + 3y + 6z - 8 = 0 $$
Using the formula:
$$ d = \frac{| -8 - 4 |}{\sqrt{2^2 + 3^2 + 6^2}} = \frac{12}{\sqrt{4+9+36}} = \frac{12}{\sqrt{49}} = \frac{12}{7} \approx 1.71 $$
Thus, the distance between the two planes is approximately 1.71 units.
Properties of Parallel Planes
Parallelism between planes follows these fundamental properties:
- Reflexive property: Every plane is parallel to itself. $$ \alpha \parallel \alpha $$
- Symmetric property: If a plane \(\alpha\) is parallel to a plane \(\beta\), then \(\beta\) is also parallel to \(\alpha\). $$ \alpha \parallel \beta \quad \Rightarrow \quad \beta \parallel \alpha $$
- Transitive property: If a plane \(\alpha\) is parallel to a plane \(\beta\), and \(\beta\) is parallel to a plane \(\gamma\), then \(\alpha\) is also parallel to \(\gamma\). $$ \alpha \parallel \beta, \quad \beta \parallel \gamma \quad \Rightarrow \quad \alpha \parallel \gamma $$
These three properties establish that parallelism between planes is an equivalence relation in geometry.
Additional Notes
Here are a few additional insights on parallel planes:
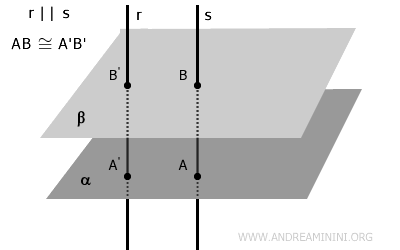
- If two planes are parallel, then any line perpendicular to one of them is also perpendicular to the other.
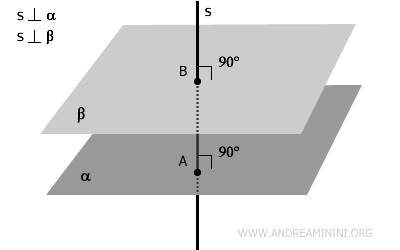
- If two parallel planes are intersected by two parallel lines—whether perpendicularly or at an angle—the segments of these lines between the planes are congruent. $$ AB \cong A'B' $$.
And so on.
