Similarity
Similarity in geometry refers to a specific relationship between shapes that maintains angle congruency and a consistent proportionality ratio, k, between corresponding sides. This relationship is achieved through the combination of a dilation and an isometry, resulting in a transformation that alters size while preserving shape.
This transformation keeps the shape intact but not its dimensions, demonstrating that two figures can be similar if they can be mapped onto each other through this process, indicating:
- Parallel corresponding sides, showing an affinity between them.
- Angles that are congruent, meaning they have the same measure.
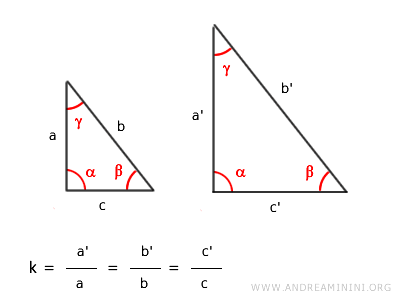
- Proportional corresponding sides with a uniform ratio of proportionality, k, across all of them.
Essentially, a figure that is similar to another retains its shape but varies in size.
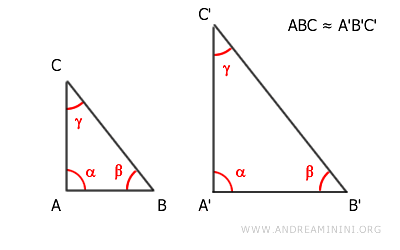
The hallmark symbol for similarity is the double tilde "≈".
To exemplify, the similarity between figures ABC and A'B'C' is denoted as:
$$ ABC ≈ A'B'C' $$
Corresponding sides and angles in similar figures are termed homologous sides and homologous angles.
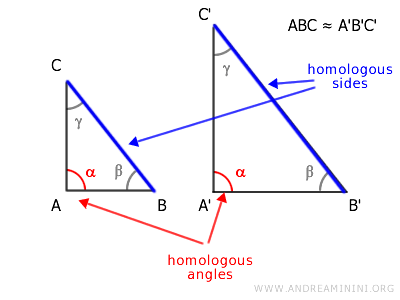
Note: In two similar triangles, corresponding sides are those positioned opposite to the congruent angles of the two triangles. In other words, corresponding sides are the sides opposite to the congruent angles.
The constant ratio of proportionality (k) for all homologous sides is referred to as the similarity ratio or scale factor.

Hence, the invariants of similarity include the angles' measures and the ratios of corresponding segments.
An everyday example of similarity is enlarging a photo to fit a larger frame, where both the original and enlarged photos share the same shape but differ in size.
Similarly, the concept of similarity extends to three-dimensional space, where solids maintain congruent corresponding angles and parallel segments with the same proportionality ratio |k|.
The Similarity Ratio
For two similar figures, the lengths of their corresponding sides are proportional, defining the similarity's scale factor.
- Enlargement (k>1)
With a similarity ratio greater than 1, the figure is scaled up by a factor of 1:k.
For example, with k=2, the figure is enlarged to twice its original size, effectively doubling all dimensions. - Isometry (k=1)
A similarity ratio of 1 results in an isometric transformation, maintaining the original size and shape.
- Reduction (0<k<1)
A similarity ratio between 0 and 1 indicates a reduction, scaling the figure down by a factor of 1:k.
For instance, if k=0.5, the figure is scaled down to half its size, or equivalently, reduced to a scale of 2:1.
Regardless of the transformation, similar figures always retain their shape.
Similar Polygons
Polygons are considered similar if their corresponding sides are in constant proportion and their angles match in measure, ensuring their shapes are congruent.
By extension, all regular polygons and circles are inherently similar to one another.
Criteria for Triangle Similarity
Specific criteria define similarity between geometric figures, particularly triangles, such as:
- AAA Criterion (Angle-Angle-Angle)
Triangles with congruent corresponding angles are similar, confirming similarity through angular congruence alone.
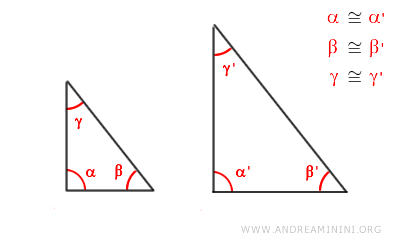
Note: In practice, verifying two congruent angles is sufficient to establish similarity between triangles, as the third angle will inherently be congruent due to the constant sum of angles in a triangle equating to 180 degrees.
- SAS Criterion (Side-Angle-Side)
Similarity is also established when two sides of a triangle are proportional to those of another, with the included angles being equal.

- SSS Criterion (Side-Side-Side)
Triangles with proportional sides are deemed similar, indicating a uniform scale factor across all corresponding sides.
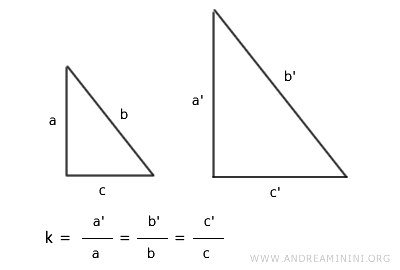
Equations of Similarity
The mathematical representation of similarity in the Cartesian plane involves a system of matrix equations, illustrating the transformation's effect on coordinates.
$$ \begin{pmatrix} x' \\ y' \end{pmatrix} = M \cdot \begin{pmatrix} x \\ y \end{pmatrix} + \begin{pmatrix} c \\ d \end{pmatrix} $$
Where M varies, depending on the nature of similarity, whether direct or inverse.
- Direct Similarity $$ M = \begin{pmatrix} a & -b \\ b &a \end{pmatrix} $$
- Inverse Similarity $$ M = \begin{pmatrix} a & b \\ b & -a \end{pmatrix} $$
Direct and inverse similarities thus follow distinct Cartesian systems, with the similarity ratio k calculated as:
$$ k = \sqrt{a^2+b^2} $$
Alternatively, similarity can be expressed through trigonometric forms, further demonstrating its flexibility in geometric transformations.
Spatial Similarity
Spatial similarity is defined in much the same way as similarity in a plane.
It is a geometric transformation that results from combining an isometry (a transformation that preserves distances, such as translations, rotations, and reflections) with a dilation (a transformation that enlarges or shrinks an object while maintaining its proportions).
Key Properties of Spatial Similarity
Spatial similarity has the following properties:
- It preserves angles between geometric elements.
- It transforms line segments into proportional segments, maintaining a constant similarity ratio.
- The similarity ratio is the same as the dilation factor and determines the scale of the transformation.
Real-World Examples of Spatial Similarity
If a structure is scaled with a similarity ratio of \( k = 2 \), all its dimensions—length, height, and depth—will double in size compared to the original.
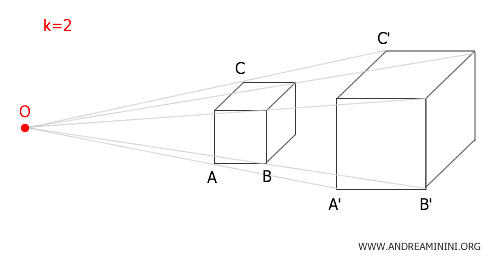
On the other hand, if a solid undergoes a transformation with a similarity ratio of \( k = \frac{1}{2} \), its edges will be half as long as those of the original, but its overall shape will remain unchanged.
Observations
Exploring the nuances of similarity, we encounter several key insights:
- Composition of Similarity
In the plane, the concept of similarity elegantly combines a dilation and an isometry, including translations, rotations, and symmetries. This integration exemplifies the seamless interplay between scaling and preserving orientation, illustrating the intricate beauty of geometric transformations.

- Congruence and Similarity
Intriguingly, congruent figures also manifest similarity. This stems from combining dilation, centered at any chosen point with a scale factor of one, with any form of isometry. This duality between congruence and similarity enriches our understanding of geometric relationships. - Interplay of Similarities
When two similarities intersect, each marked by its distinct scale ratios k1 and k2, they merge into a singular similarity. This phenomenon underscores the elegance of geometric transformations, highlighting how combined scale ratios reflect the essence of both contributing similarities. - The Anchor Point of Similarity
Every similarity in the plane, except for translations, is characterized by a "center of similarity". This fixed point acts as a steadfast reference throughout the transformation, marking the continuity and coherence of the process. - Navigating Similarities
The distinction between direct and indirect similarities is determined by their orientation relative to the plane. Direct similarities conserve orientation, while indirect similarities alter it, offering a deeper layer of understanding in geometric transformations. - Area and Similarity
The proportional relationship between the areas of similar figures directly correlates to the square of their similarity ratio k. This principle elegantly demonstrates the scalable nature of shapes and their geometric properties in the plane. - Volume and Similarity
In the realm of three dimensions, the volume ratio of similar solids is proportionate to the cube of their similarity ratio k, extending the principles of similarity to volumetric considerations and enhancing our comprehension of spatial geometry.
Through this deeper dive into similarity, we uncover the intricate layers and principles that define geometric transformations, providing a comprehensive view of spatial relationships.
