Isometry
Isometry is a geometric transformation that preserves the distances between points.
In simpler terms, if two points have a certain distance between them before the transformation, that distance remains unchanged afterward.
This means that every isometry transforms a shape into a congruent shape.
For example, the first triangle is rotated 90° clockwise and shifted to the right. The distances between points A, B, and C remain the same.
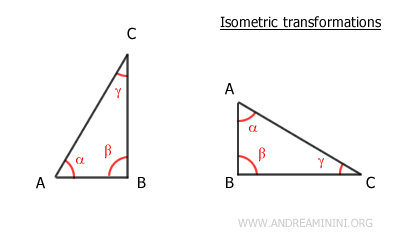
Therefore, rotation and translation are both examples of isometric transformations.
Additionally, both triangles are congruent because their side lengths stay the same.
The term 'isometric' is often used interchangeably with 'congruent'. The word has Greek origins, where 'isos' means 'same' and 'metros' means 'measure'. For example, the two triangles mentioned earlier are congruent because their sides and angles remain congruent even after rotation and translation.
Transformations that result in an isometry are called isometric transformations or rigid transformations (or rigid motions) because they do not alter the shape or size of objects.
Shapes that remain unchanged under isometry are called isometric figures, as they retain the same measurements.
Types of Isometries
The main types of isometric transformations are:
- Translation
Every point is moved by a fixed amount in a specific direction.
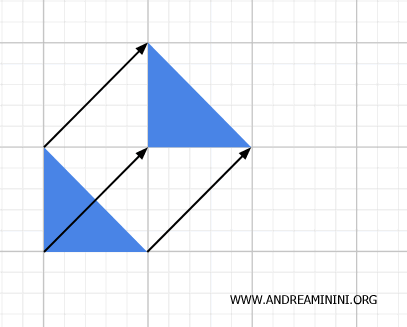
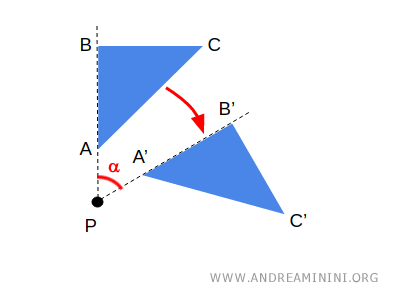
- Rotation
Every point rotates around a fixed point known as the center of rotation.
- Reflection
Every point is reflected across a line, called the axis of reflection.

- Central Symmetry
Every point is mapped to its diametrically opposite point relative to a central point.
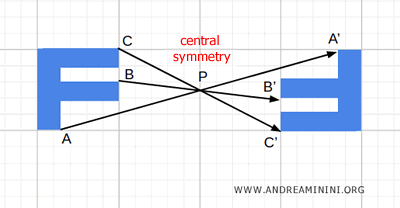
Compositions of Isometries
When you apply two or more isometric transformations in a specific sequence to a geometric figure, the final result can be described as a composition of isometries.
For example, you can translate a triangle 10 cm along a horizontal line (translation) and then rotate it 180° (rotation).

It's important to note that the composition of two isometries is still an isometry.
Since each isometry individually preserves distances and shapes, a composition of isometries will also retain these properties.
In other words, a figure subjected to a composition of isometries maintains its original shape and size.
Note: However, the composition of isometries is not always commutative. This means the order in which you perform the geometric transformations can affect the final result. For example, a rotation followed by a translation yields a different result than a translation followed by a rotation.
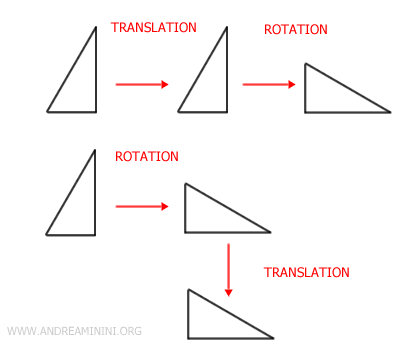
Isometric compositions can have specific names depending on the transformations combined. For example:
- Glide Reflection
The combination of a reflection and a translation is known as a "glide reflection". - Rototranslation
The combination of a rotation and a translation is referred to as a "rototranslation".
Direct and Indirect Isometries
Isometries can be classified as either direct or indirect.
- Direct Isometries
Direct isometries preserve the orientation of a figure. In other words, when a figure undergoes a direct isometry, its clockwise or counterclockwise orientation remains unchanged. Examples of direct isometries include translations and rotations. To illustrate, these two flags can be perfectly aligned using a combination of two rigid motions (rotation + translation). This is an example of a direct isometry.

- Indirect Isometries
Indirect isometries reverse the orientation of a figure and include only reflections, such as axial symmetry. This means that a figure transformed by an indirect isometry switches from clockwise to counterclockwise orientation, or vice versa. For instance, these flags can only be perfectly aligned after a reflection, as the transformation "mirrors" the figure. This is an example of an indirect isometry.
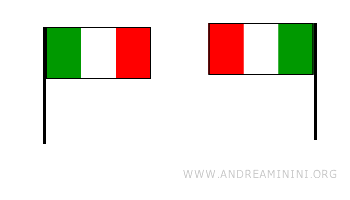
Note: A common mistake is assuming that an indirect isometry must always result from two rigid motions, whereas in reality, a single reflection is enough to reverse orientation. Therefore, the number of geometric transformations in a composition does not determine whether a figure is direct or indirect, only the overall effect on the figure's orientation matters.
Invariants in Isometry
In an isometry, certain characteristics of a shape may change, such as its position, while others remain unchanged.
The features that do not change are known as "invariants" in isometry.

The main invariant properties during an isometry are:
- Distance between points
In isometries, the distance between points remains unchanged. This means that, for example, if the distance between two points A and B is 5 cm in the original figure, it will remain the same in the resulting figure after an isometric transformation. - Length of segments
The length of segments does not change between the original figure and its image. For instance, the segments AB, BC, and AB have the same length in both triangles. - Angle measures
The measure of the angles remains constant after an isometry. For example, the angles α, β, and γ of the triangle maintain the same measure after the isometric transformation. - Area of the figure
The total area of the figure does not change. For example, the area of the triangle remains the same after the isometric transformation. - Shape and size
In an isometry, the shape and size of the geometric figure do not change. These properties are invariant. - Alignment of points
If two or more points are aligned in the original figure, they will also be aligned in its image. For example, points A and B are aligned in both the original triangle and the resulting triangle after the transformation. - Parallelism of lines
Lines that are parallel in the original figure remain parallel in the image. - Perpendicularity of lines
Lines that are perpendicular in the original figure maintain this characteristic in their image as well. For example, segments AB and BC are perpendicular in both the first triangle (original) and the second triangle (isometric image).
Isometries in Space
Isometries in space are transformations that preserve the distances between corresponding points, keeping the shape and size of solids unchanged while altering only their position and/or orientation.
In two-dimensional geometry, isometric transformations include translations, rotations, and reflections—the same applies in three-dimensional space.
Isometries in space share the same fundamental properties as those in the plane:
- They preserve the distances between corresponding points.
- They transform each segment into a congruent segment of the same length.
- They maintain the angles between segments and between planes.
Two solids are considered congruent if they have the following identical characteristics: corresponding edges of equal length, corresponding angles of the same measure, congruent corresponding edges and faces, and equal corresponding dihedral angles.
- Directly congruent solids
Two solid figures are directly congruent if they can be perfectly aligned using rigid motions such as translations and rotations, without requiring reflection.Example: Two identical cubes that can be aligned through translation and/or rotation.
- Inversely congruent solids
Two solid figures are inversely congruent if they can be perfectly aligned only by introducing a reflection.Example: A right shoe and a left shoe. They have the same shape but are mirror images of each other, requiring reflection to match.
Additional Observations
Here are some additional observations and side notes on isometries:
- Isometries are congruences
Isometries are geometric transformations that produce congruent figures because they preserve segment lengths and angle measures. As a result, the transformed figures are point-for-point superimposable onto the original figures after some rigid motions, fulfilling the definition of congruence in Euclidean geometry. - Isometries are a specific type of similarity
Isometries are similarities with a similarity ratio of 1. They satisfy all the properties of similarities:- they preserve the parallelism between segments,
- corresponding angles are congruent, and
- corresponding segments are congruent.
And so on.
