Trivial Vector Space
The trivial vector space (or null vector space) contains only the zero element. $$ V = \{ \vec{0} \} $$
It's the smallest possible vector space.
Is the null vector space an empty set? No, the null vector space is not the empty set (V = {}). This is because an empty set does not satisfy the commutative property of vector spaces. Therefore, an empty set is not a vector space.
Properties of the Null Vector Space
Any vector v∈V multiplied by zero results in the null space 0v.
Similarly, any scalar α∈K multiplied by the null space 0v results in the null space itself.
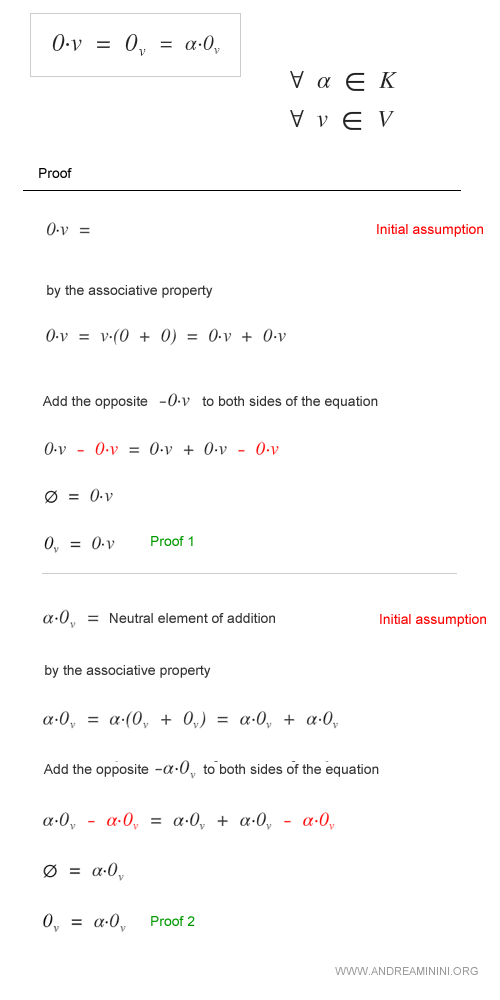
And so on.
