Affine Transformations

An affine transformation (or affinity) is a geometric transformation that preserves the parallelism between lines.

An affinity is a type of function that maps points, lines, and shapes from one plane or space to another, while keeping parallel lines parallel.

Affine transformations can either be isometric, preserving lengths and angles, or non-isometric, where these measurements may change.

However, what remains constant is the parallelism between lines.

A Practical Example

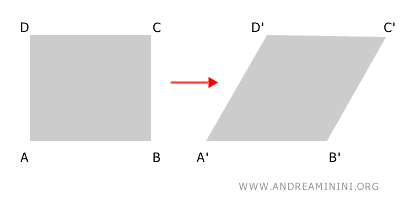
Example 1: Non-Isometric Affinity

This geometric transformation is affine because it preserves the parallelism between lines.

It’s a non-isometric affine transformation since it doesn’t preserve congruence; the angles and lengths of segments change.

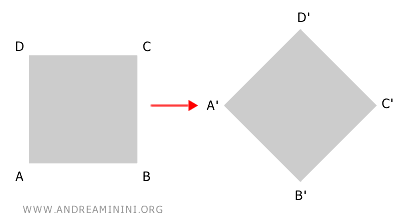
Example 2: Isometric Affinity

This is an example of an isometric affinity, which involves a combination of translation and rotation.

In this case, both the parallelism between lines and the congruence of corresponding lengths and angles are preserved.

And so on.