Associated Angles α and π/2 minus α in Trigonometry

In trigonometry, the associated angles alpha (α) and π/2-α allow us to use these transformation formulas: $$\sin( \frac{ \pi }{2} - \alpha) = \cos(\alpha)$$ $$\cos( \frac{ \pi }{2} - \alpha) = \sin(\alpha)$$ $$\tan( \frac{ \pi }{2} - \alpha) = \cot(\alpha)$$ $$\cot( \frac{ \pi }{2} - \alpha) = \tan(\alpha)$$

Angles α and π/2-α are associated angles, meaning they yield the same absolute value in trigonometric functions such as sine, cosine, tangent, and cotangent.

Proof and Explanation

Let’s examine an angle α and the angle π/2-α on a unit circle.

Angles α and π/2-α are complementary angles because their sum equals 90° (π/2), a right angle.

$$\alpha + (\frac{\pi}{2} - \alpha) = \frac{\pi}{2}$$

We can construct two right triangles, OAB and OCD, on the unit circle.

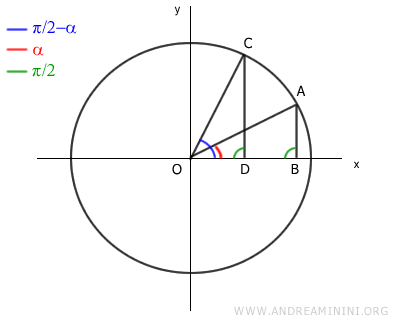
In both triangles, we already know two of the angles.

Since the sum of the angles in any triangle is π (180°), we can calculate the remaining angle in each triangle.

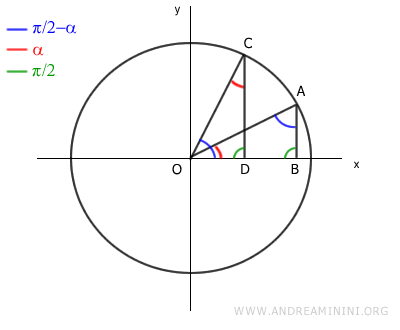
Note. In the first triangle, OAB, one angle is acute (α) and the other is a right angle (π/2 or 90°). Thus, the remaining angle must be π/2-α, since the sum of the angles is 180° (π). In the second triangle, OCD, one angle is π/2-α, and the right angle is π/2 (90°), leaving the other acute angle to be α, again due to the sum of the angles being 180° (π).

Therefore, the two triangles, OAB and OCD, are congruent because they share the same angles and hypotenuse.

This means the corresponding sides of the triangles must also have the same lengths.

The segment OB (cosine of α) is equal to the segment CD (sine of π/2-α).

Therefore, the sine of π/2-α is equal to the cosine of α.

$$\sin ( \frac{\pi}{2} - \alpha ) = \cos \alpha$$

The segment AB (sine of α) is equal to the segment OD (cosine of π/2-α).

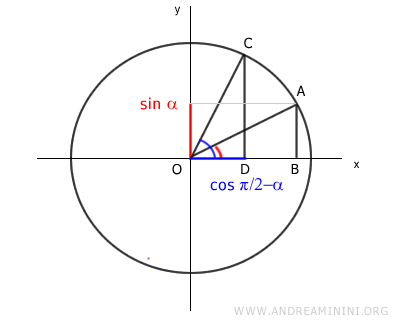
Thus, the cosine of π/2-α is equal to the sine of α.

$$\cos ( \frac{\pi}{2} - \alpha ) = \sin \alpha$$

Once we know the transformation formulas for sine and cosine, we can also derive the corresponding formulas for tangent and cotangent.

The tangent is the ratio of sine to cosine.

$$\tan ( \frac{\pi}{2} - \alpha ) = \frac{ \sin (\frac{\pi}{2} - \alpha) }{ \cos (\frac{\pi}{2} - \alpha) }$$

Knowing that sin(π/2-α) = cos(α) and cos(π/2-α) = sin(α), we get:

$$\tan ( \frac{\pi}{2} - \alpha ) = \frac{ \cos \alpha }{ \sin \alpha } = \cot \alpha$$

So, the tangent of π/2-α is equal to the cotangent of α.

The cotangent is the ratio of cosine to sine.

$$\cot ( \frac{\pi}{2} - \alpha ) = \frac{ \cos (\frac{\pi}{2} - \alpha) }{ \sin (\frac{\pi}{2} - \alpha) }$$

Again, knowing that sin(π/2-α) = cos(α) and cos(π/2-α) = sin(α), we get:

$$\cot ( \frac{\pi}{2} - \alpha ) = \frac{ \sin \alpha }{ \cos \alpha } = \tan \alpha$$

Thus, the cotangent of π/2-α is equal to the tangent of α.

Practical Example

Let’s calculate the sine of 30°.

$$\sin 30°$$

We can rewrite this as: 90° - 60°

$$\sin (90° - 60°)$$

In radians, this becomes:

$$\sin (\frac{\pi}{2} - \frac{\pi}{3} )$$

Here, π/2-α and α are associated angles, where α = π/3 (or 60°).

$$\sin( \frac{\pi }{2} - \alpha ) = \cos(\alpha)$$

$$\sin( \frac{\pi }{2} - \frac{\pi}{3} ) = \cos( \frac{\pi}{3} )$$

So, the sine of 30° is equal to the cosine of 60°.

Since the cosine of 60° is 1/2:

$$\sin( \frac{\pi }{2} - \frac{\pi}{3} ) = \cos( \frac{\pi}{3} ) = \frac{1}{2}$$

Therefore, the sine of 30° is 1/2.

$$\sin 30° = \frac{1}{2}$$

And so on.