Cosecant
What is the Cosecant?
In trigonometry, the cosecant is a function that associates an angle α with the reciprocal of its sine. It is represented by the symbol csc or cosec. $$ \csc \alpha = \frac{1}{\sin \alpha} $$
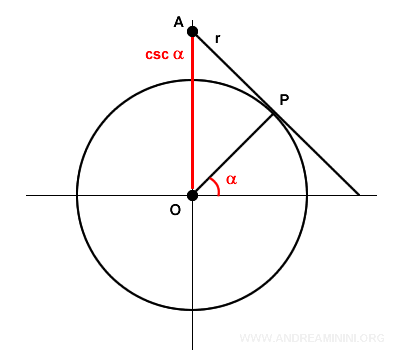
Geometrically, the cosecant (OA) can be visualized as the intersection of the line (r) tangent to point P on the unit circle and the vertical y-axis.
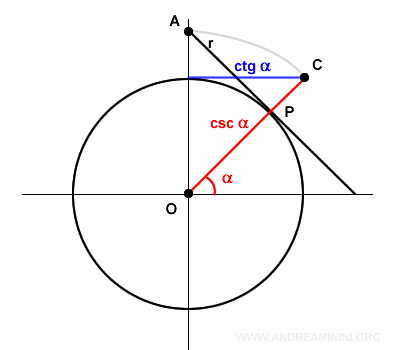
The cosecant can also be understood as the line segment OC, which starts at the origin O, passes through point P, and intersects the cotangent at point C.
The cosecant function is defined for all real numbers R except at points kπ where k is an integer, as the sine function equals zero at these points.
$$ \sec \alpha \ : \ R - k \cdot \pi \rightarrow R - (-1,1) \ \ \ \ \ \ \ \ k \in Z $$
The codomain is the set of all real numbers R, excluding the interval (-1,1).

From a mathematical perspective, the secant is an odd function since f(x) = -f(-x).
It is also a periodic function, which can be examined within the interval (0, 2π).
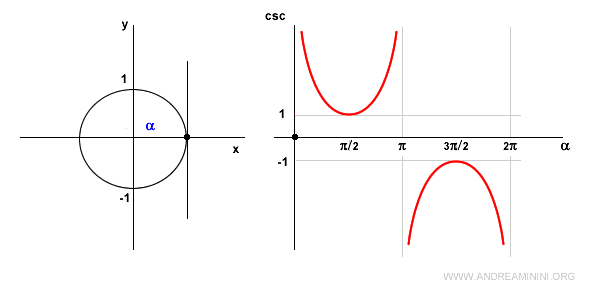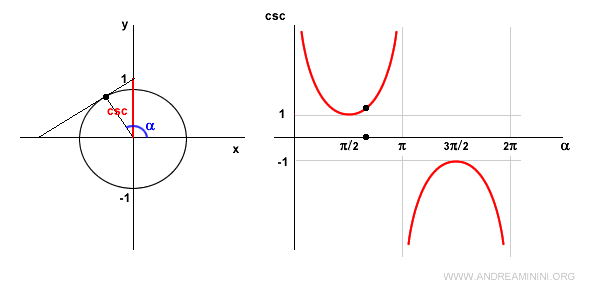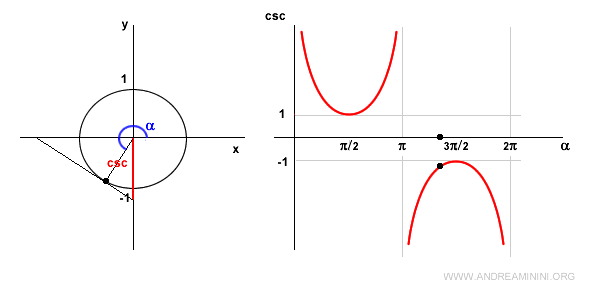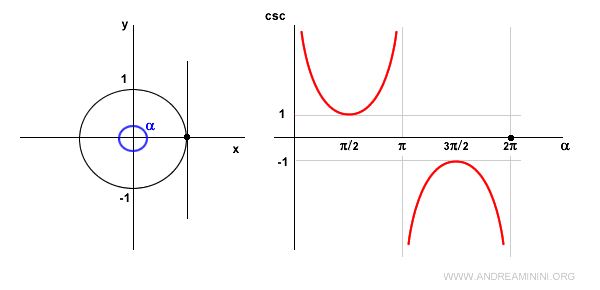
Note: The cosecant function is undefined at points kπ where k is an integer. At these points, it exhibits a vertical asymptote.
Here are some key values of the secant that are useful to remember:
| Degrees | Radians | Secant |
|---|---|---|
| 0° | 0 | Undefined |
| 30° | π/6 | 2 |
| 45° | π/4 | √2 |
| 60° | π/3 | (2√3)/3 |
| 90° | π/2 | 1 |
| 180° | π | Undefined |
| 270° | 3/2π | -1 |
| 360° | 2π | Undefined |
The Proof
Triangle OPB is similar to triangle OAP because they share the same angles.
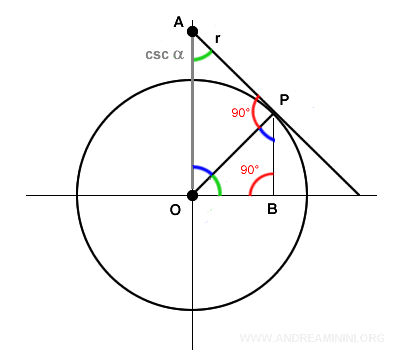
This similarity allows us to write the following proportion:
$$ \overline{OP} \ : \overline{PB} = \overline{OA} \ : \overline{OP} $$
or
$$ \frac{ \overline{OP} } { \overline{PB} } = \frac{ \overline{OA} } { \overline{OP} } $$
The segment PB represents the sine of the angle α:
$$ \frac{ \overline{OP} } { \sin \alpha } = \frac{ \overline{OA} } { \overline{OP} } $$
Since the radius of the unit circle is 1, we substitute OP = 1:
$$ \frac{ 1 } { \sin \alpha } = \frac{ \overline{OA} } { 1 } $$
$$ \frac{ 1 } { \sin \alpha } = \overline{OA} $$
The segment OA is the cosecant of angle α:
$$ \frac{ 1 } { \cos \alpha } = \csc \alpha $$
Thus, we obtain the formula for the secant as the reciprocal of the cosine.
Alternative Proof: Triangles ODC and OEP are similar since they have identical angles.
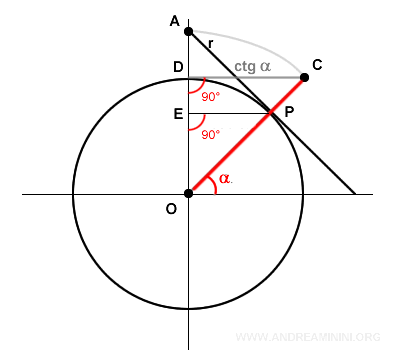
Therefore, there is a proportional relationship between the legs and the hypotenuses: $$ \frac{ \overline{OE} } { \overline{OP} } = \frac{ \overline{OD} } { \overline{OC} } $$ Knowing that OC is the cosecant: $$ \frac{ \overline{OE} } { \overline{OP} } = \frac{ \overline{OD} } { \csc \alpha } $$ The segment OE represents the sine: $$ \frac{ \sin \alpha } { \overline{OP} } = \frac{ \overline{OD} } { \csc \alpha } $$ Since both OP and OD are radii of the unit circle, OP = OD = 1. $$ \frac{ \sin \alpha } { 1 } = \frac{ 1 } { \csc \alpha } $$ Highlighting the cosecant gives us the formula we set out to prove: $$ \csc \alpha = \frac{ 1 } { \sin \alpha } $$
Other Cosecant Formulas
The cosecant of an angle α can also be calculated using the tangent of the angle: $$ \csc \alpha = \sqrt{1 + \cot^2 \alpha } $$
Proof
The radius, cosecant, and cotangent form a right triangle, with the cosecant as the hypotenuse.
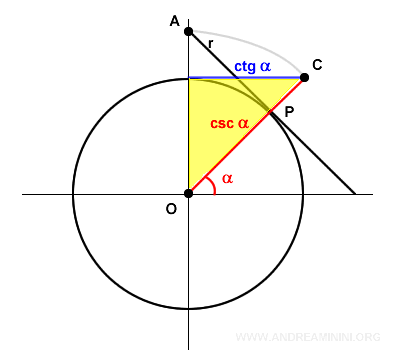
Thus, we can apply the Pythagorean theorem in terms of the cotangent:
$$ \csc \alpha = \sqrt{1^2 + \cot^2 \alpha } $$
$$ \csc \alpha = \sqrt{1 + \cot^2 \alpha } $$
It can also be expressed using the sine and cosine functions since cot α = cos α / sin α:
$$ \csc \alpha = \sqrt{1 + \frac{\cos^2 \alpha}{\sin^2 \alpha} } $$
The Graph of the Cosecant
When the angle is zero (α = 0), the secant is undefined because sin 0 = 0.
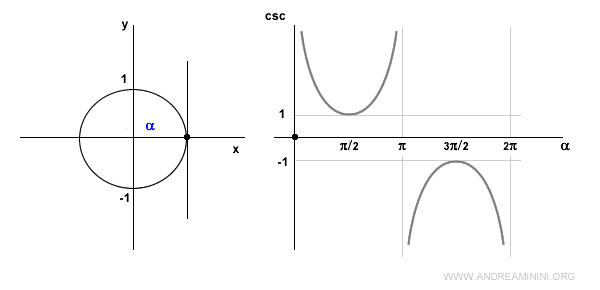
In the first quadrant, when α is positive, the sine is positive, making the cosecant positive and decreasing.
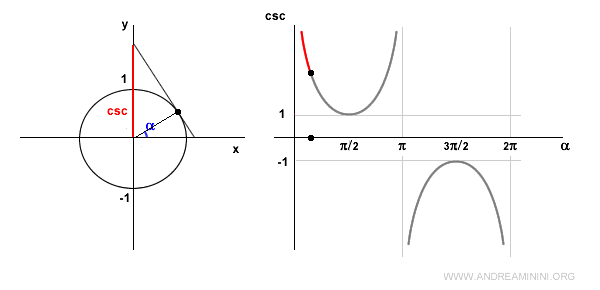
At α = π/2 (90°), the cosecant equals 1 since sin(π/2) = 1.
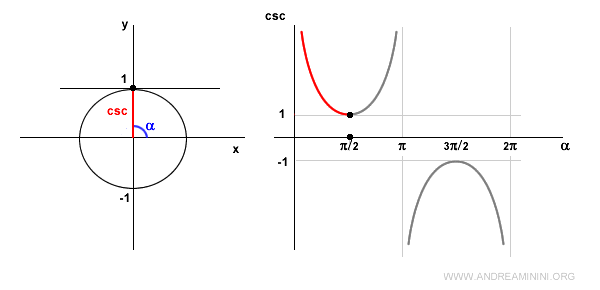
In the second quadrant, the sine remains positive, so the secant is positive but increasing.
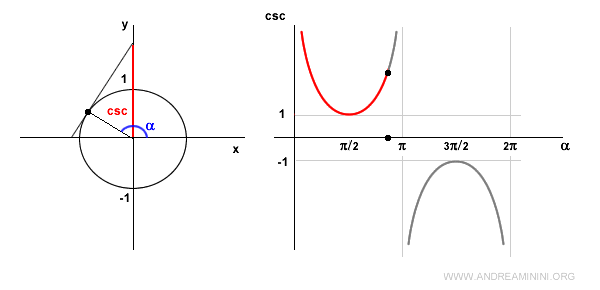
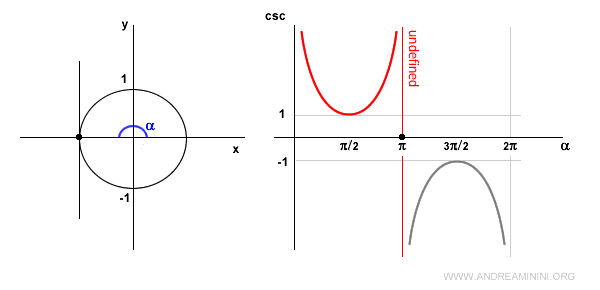
At α = π (180°), the cosecant is undefined because sin(π) = 0, resulting in an undefined ratio (sec(π) = 1/0).
In this scenario, the tangent line at the point is parallel to the y-axis, meaning no intersection exists between the two lines.
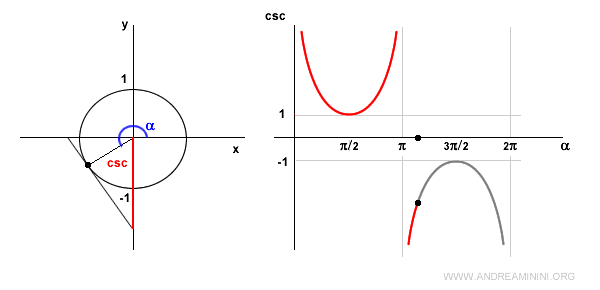
In the third quadrant, where α is negative, the sine is also negative.
Thus, the secant is negative and increasing.
At α = 3π/2 (270°), the sine equals -1.
Therefore, the cosecant is -1.
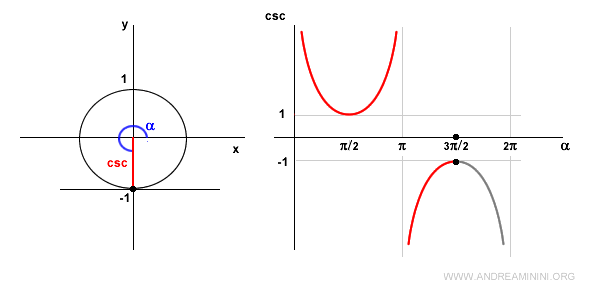
In the fourth quadrant, where the sine is negative, the cosecant is likewise negative and decreasing.
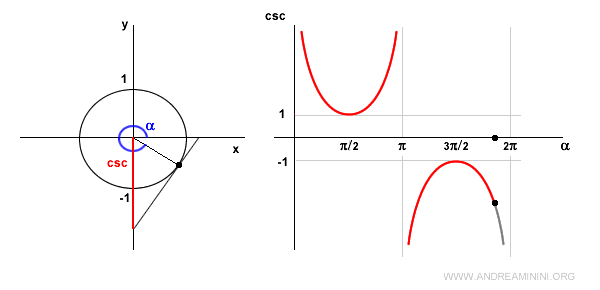
At α = 2π, we return to the same situation as with an angle of zero (α = 0).
The secant is undefined since the sine function equals zero, as sin(2π) = sin(0) = 0.
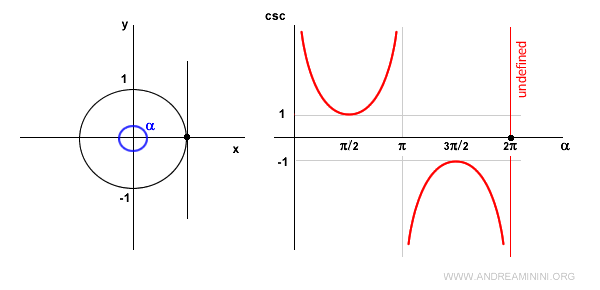
Since it is a periodic function, this cycle repeats indefinitely.
