Associated Angles: α and π-α in Trigonometry

In trigonometry, the associated angles alpha (α) and π-α allow us to apply the following transformation formulas: $$ \sin(\pi-\alpha) = \sin(\alpha) $$ $$ \cos(\pi-\alpha) = -\cos(\alpha) $$ $$ -\tan(\pi-\alpha) = \tan(\alpha) $$ $$ -\cot(\pi-\alpha) = \cot(\alpha) $$

Since they are associated angles, α and π-α share the same absolute values for the trigonometric functions sine, cosine, tangent, and cotangent.

Proof and Explanation

Let’s consider an angle α and the angle π-α on a unit circle.

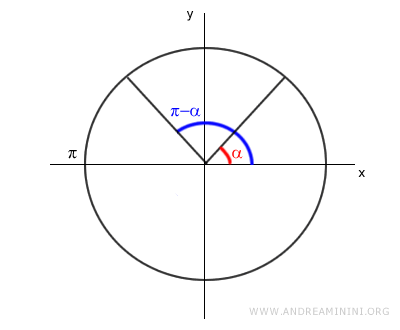
The angles α and π-α are supplementary angles because their sum equals 180° (π).

$$ \alpha + (\pi - \alpha) = \pi $$

Thus, the two right triangles OAB and OCD are congruent because they have the same hypotenuse (OA=OC), the same acute angle (α), and of course, a right angle (90°).

Both triangles OAB and OCD have identical angles and side lengths.

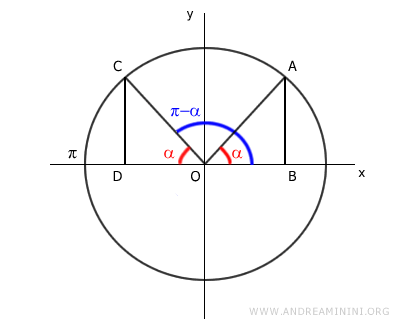
Therefore, the supplementary angles α and π-α have the same value for the sine function (AB=CD).

$$ \sin \alpha = \sin (\pi - \alpha) $$

This is easily shown graphically.

The angles α and π-α also share the same absolute value for the cosine function (OB=OD).

However, the cosine values are opposite, as OD lies on the negative x-axis, while OB is on the positive x-axis.

$$ \cos \alpha = - \cos (\pi - \alpha) $$

Multiplying both sides of the equation by -1:

$$ -(1) \cdot \cos \alpha = (-1) \cdot (- \cos (\pi - \alpha)) $$

$$ - \cos \alpha = \cos (\pi - \alpha) $$

This can also be illustrated graphically.

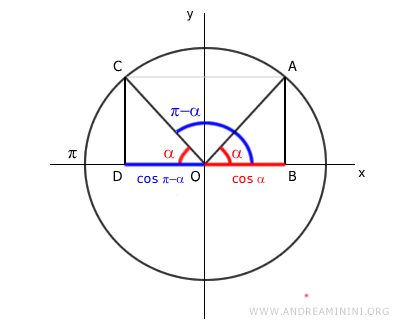
Thus, the cosine of the associated angles α and π-α has opposite signs.

Once we know the transformation formulas for sine and cosine, we can derive those for tangent and cotangent.

The tangent is the ratio between sine and cosine.

$$ \tan (\pi - \alpha) = \frac{\sin (\pi - \alpha)}{\cos (\pi - \alpha)} $$

Knowing that sin(π-α) = sin(α) and cos(π-α) = -cos(α), we get:

$$ \tan (\pi - \alpha) = \frac{\sin \alpha}{- \cos \alpha} = - \tan \alpha $$

So, the tangent of the angle π-α is the negative of the tangent of α.

The cotangent is the ratio between cosine and sine.

$$ \cot (\pi - \alpha) = \frac{\cos (\pi - \alpha)}{\sin (\pi - \alpha)} $$

Knowing that sin(π-α) = sin(α) and cos(π-α) = -cos(α), we get:

$$ \cot (\pi - \alpha) = \frac{- \cos \alpha}{\sin \alpha} = - \cot \alpha $$

Therefore, the cotangent of π-α is the negative of the cotangent of α.

In conclusion, the tangent and cotangent of the associated angles α and π-α have opposite signs.

A Practical Example

Let’s calculate the sine of 150°.

$$ \sin 150° $$

We can rewrite this as 180° - 30°:

$$ \sin 150° = \sin (180° - 30°) $$

In radians, this becomes:

$$ \sin 150° = \sin \left( \pi - \frac{\pi}{6} \right) $$

The angles π-α and α are associated angles, where α = π/6 (or 30°).

$$ \sin(\pi - \alpha) = \sin(\alpha) $$

Thus, the sine of 150° is equal to the sine of 30°:

$$ \sin 150° = \sin \left( \pi - \frac{\pi}{6} \right) = \sin \left( \frac{\pi}{6} \right) $$

Since the sine of 30° is 1/2, the sine of 150° is also 1/2.

$$ \sin 150° = \frac{1}{2} $$

And so on.