The Parallel Postulate

The parallel postulate, also known as Euclid's fifth postulate, states:

Given a line r and a point P not on the line, there exists exactly one line parallel to r that passes through point P.

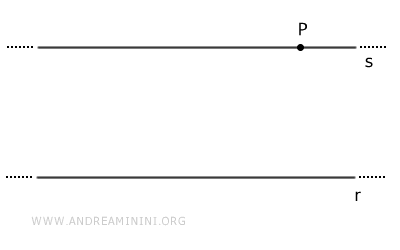
This is considered a postulate because the uniqueness of the parallel line is not derived from other theorems or properties of the line. It is accepted as a fundamental truth.

However, the existence of a line parallel to r passing through point P can be demonstrated using the parallel lines theorem by finding a pair of congruent alternate interior angles α≅β.

Note: This postulate is known as Euclid's fifth postulate. However, its formulation is attributed to Proclus, a Byzantine mathematician and philosopher who lived in the 5th century AD.

The Proof

Consider a line r and a point P in the plane that does not lie on the line.

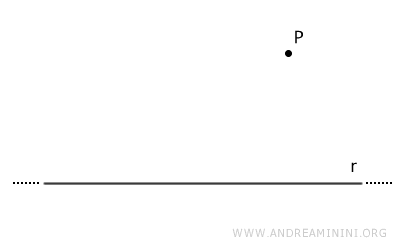
Choose a point P' on the line.

Then draw a line t passing through points P' and P.

Line t forms an interior angle alpha (α) with line r.

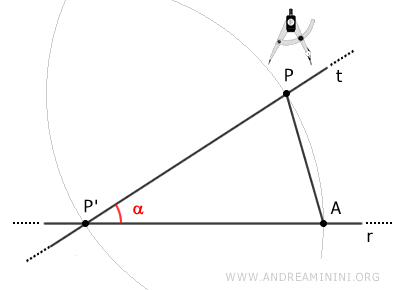
Draw an arc centered at P' with radius PP' that intersects line r at point A.

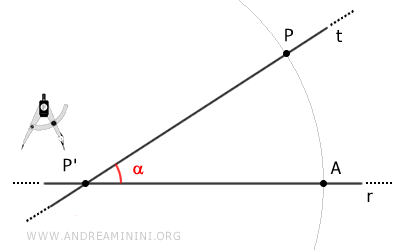
Center the compass on point P and with the same radius (PP'), draw a second arc that passes through point P'.

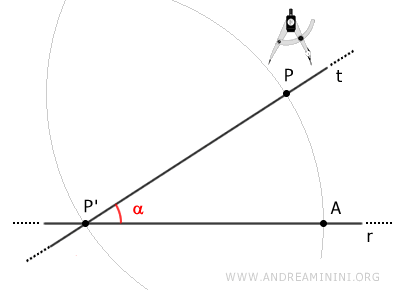
Connect points A and P with a segment AP.

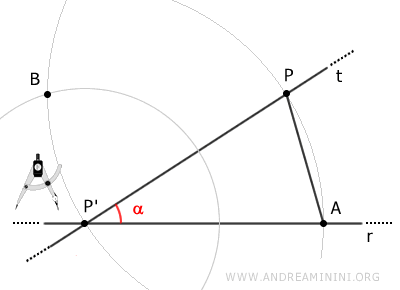
Draw a third arc centered at P' with radius AP that intersects the second arc at point B.

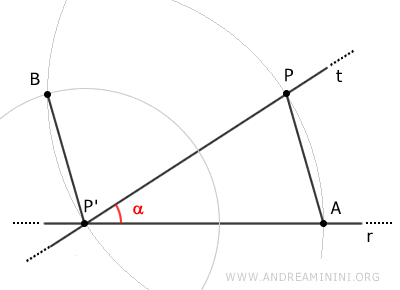
Then connect points P' and B with segment BP'.

Finally, draw a line s passing through points B and P.

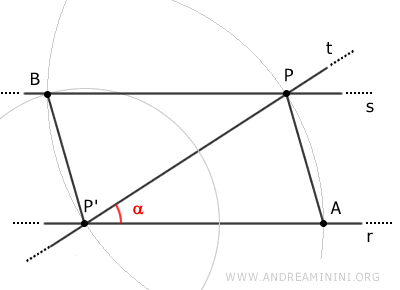
Two triangles, APP' and BPP', are formed between lines r and s.

According to the third criterion for triangle congruence, the triangles APP' and BPP' are congruent because they have sides of equal length.

$$ APP' \cong BPP' $$

Since they are congruent, triangles APP' and BPP' have congruent angles in corresponding positions.

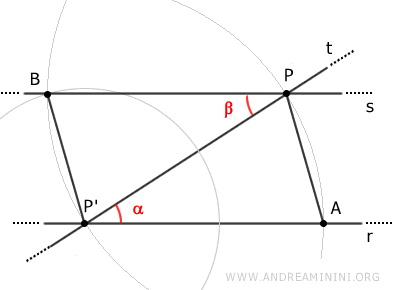
Therefore, angle alpha is congruent to angle beta.

$$ \alpha \cong \beta $$

Angles alpha and beta are congruent alternate interior angles (α≅β).

Thus, by the parallel lines theorem, lines r and s are parallel.

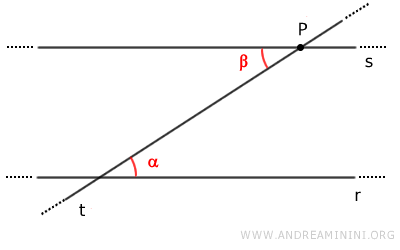
This demonstrates the existence of line s parallel to line r passing through point P

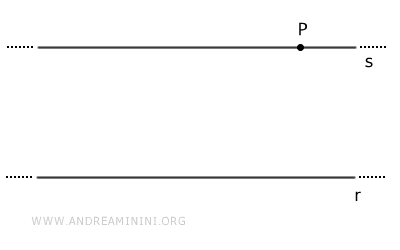
Note: As mentioned earlier, the uniqueness of the parallel line s cannot be proven through other properties. Therefore, it is accepted as a postulate.

Why can't the uniqueness be proven?

For centuries, mathematicians attempted to derive the fifth postulate from the other four, believing it to be a consequence rather than a foundational assumption. However, all such efforts failed - the postulate is logically independent.

In trying to prove that exactly one parallel can be drawn through a point external to a given line - as Euclid claimed and intuition seemed to support - mathematicians also explored what would happen if this rule were altered.

Surprisingly, instead of encountering contradictions, they uncovered entirely consistent alternative geometries.

These discoveries, made in the 19th century, led to the development of non-Euclidean geometries, each based on a different version of the parallel postulate:

• Hyperbolic geometry (Lobachevsky, Bolyai): Through a point not on a given line, infinitely many parallels can be drawn.
  
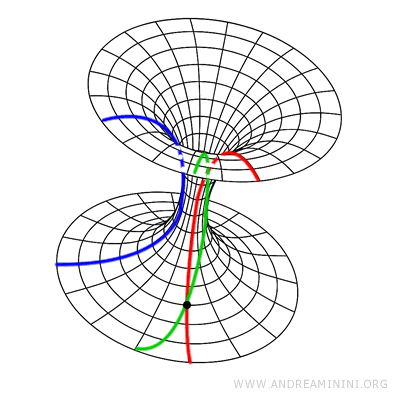
• Elliptic geometry (Riemann): No parallels exist through a point outside a given line. For example, in spherical geometry, there are no parallel lines, since all “lines” are great circles - and any two great circles always intersect in exactly two antipodal points.
  

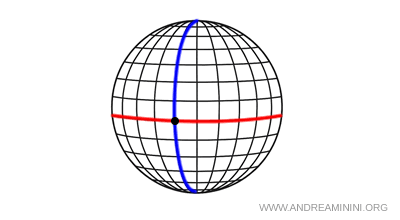
The emergence of non-Euclidean geometries demonstrated that the concept of space is not absolute. Space can be modeled in fundamentally different yet equally valid ways, with profound implications for both mathematics and physics.

Put simply, Euclid’s fifth postulate marks the turning point where we realize that geometry is not uniquely determined; instead, multiple consistent geometries are possible, and Euclidean geometry is just one special case among them.

And so forth.