Trigonometric Equation sin(α) = -cos(α')

The trigonometric equation $$ \sin \alpha = - \cos \alpha' $$ can be solved using the equivalent formula $$ \sin \alpha = \sin (\alpha' - \frac{\pi}{2}) $$. The solutions are: $$ \alpha = (\alpha' - \frac{\pi}{2}) + 2k \pi \ \text{or} \ \alpha + (\alpha' - \frac{\pi}{2}) = \pi + 2k \pi $$

An Example

Let’s solve the trigonometric equation:

$$ \sin(\frac{1}{2}x) = -\cos(\frac{1}{4}x) $$

We can rewrite -cos(1/4x) as a sine function: sin(1/4x - π/2).

$$ \sin(\frac{1}{2}x) = \sin(\frac{1}{4}x - \frac{\pi}{2}) $$

This transforms the equation into the form sin(α) = sin(α'), where:

$$ \alpha = \frac{1}{2}x $$

$$ \alpha' = \frac{1}{4}x - \frac{\pi}{2} $$

Using the formula for solving equations of the type sin(α) = sin(α'), the solutions are:

$$ \alpha = \alpha' + 2k \pi \ \text{or} \ \alpha + \alpha' = \pi + 2k \pi $$

where k is any integer.

Substituting the expressions for α and α':

$$ \frac{1}{2}x = (\frac{1}{4}x - \frac{\pi}{2}) + 2k \pi \ \text{or} \ \frac{1}{2}x + (\frac{1}{4}x - \frac{\pi}{2}) = \pi + 2k \pi $$

Let’s simplify to isolate x:

$$ \frac{1}{2}x - \frac{1}{4}x = -\frac{\pi}{2} + 2k \pi \ \text{or} \ \frac{3}{4}x = \frac{\pi}{2} + \pi + 2k \pi $$

$$ \frac{1}{4}x = -\frac{\pi}{2} + 2k \pi \ \text{or} \ \frac{3}{4}x = \frac{3}{2} \pi + 2k \pi $$

$$ x = 4(-\frac{\pi}{2} + 2k \pi) \ \text{or} \ x = \frac{4}{3}(\frac{3}{2} \pi + 2k \pi) $$

$$ x = -2\pi + 8k \pi \ \text{or} \ x = 2\pi + \frac{8}{3}k \pi $$

This gives all the solutions to the equation sin(x) = sin(y).

Now, let’s verify these solutions for specific values of k.

Verification for k = 0

$$ x = -2\pi + 8k \pi \ \text{or} \ x = 2\pi + \frac{8}{3}k \pi $$

$$ x = -2\pi + 8(0)\pi \ \text{or} \ x = 2\pi + \frac{8}{3}(0)\pi $$

$$ x = -2\pi \ \text{or} \ x = 2\pi $$

Therefore, one solution is x = -2π, and another is x = 2π.

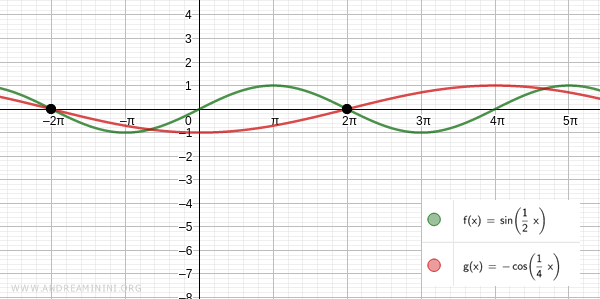
Verification for k = 1

$$ x = -2\pi + 8k \pi \ \text{or} \ x = 2\pi + \frac{8}{3}k \pi $$

$$ x = -2\pi + 8(1)\pi \ \text{or} \ x = 2\pi + \frac{8}{3}(1)\pi $$

$$ x = 6\pi \ \text{or} \ x = \frac{14}{3}\pi $$

Thus, one solution is x = 6π, and another is x = 14/3π.

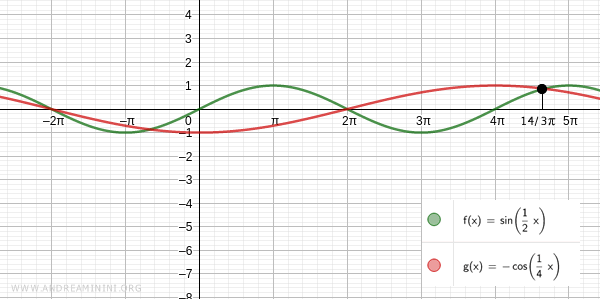
Verification for k = -1

$$ x = -2\pi + 8k \pi \ \text{or} \ x = 2\pi + \frac{8}{3}k \pi $$

$$ x = -2\pi + 8(-1)\pi \ \text{or} \ x = 2\pi + \frac{8}{3}(-1)\pi $$

$$ x = -10\pi \ \text{or} \ x = -\frac{2}{3}\pi $$

Hence, one solution is x = -10π, and another is x = -2/3π.

By varying k, we can generate all the infinite solutions to the equation.

The Proof

The cosine function:

$$ \cos \alpha $$

is closely linked to the sine function, as sine and cosine are phase-shifted by 90° (π/2).

We can express cosine as an equivalent sine function using an associated angle (π/2 - α):

$$ \cos \alpha = \sin (\frac{\pi}{2} - \alpha) $$

Since sine and cosine are out of phase by 90° (π/2), the trigonometric equation:

$$ \sin \alpha = -\cos \alpha' $$

can be rewritten as:

$$ \sin \alpha = -\sin(\frac{\pi}{2} - \alpha') $$

Using the odd symmetry of sine (-f(x) = f(-x)), we can move the negative sign into the argument:

$$ \sin \alpha = \sin(-\frac{\pi}{2} + \alpha') $$

or equivalently:

$$ \sin \alpha = \sin(\alpha' - \frac{\pi}{2}) $$

This transforms the equation into the standard form sin(α) = sin(β), whose solutions are:

$$ \alpha = \beta + 2k \pi \ \text{or} \ \alpha + \beta = \pi + 2k \pi $$

Here, β = α' - π/2:

$$ \alpha = (\alpha' - \frac{\pi}{2}) + 2k \pi \ \text{or} \ \alpha + (\alpha' - \frac{\pi}{2}) = \pi + 2k \pi $$

This completes the proof.