Difference Between Interior and Exterior Angles

Interior and exterior angles are fundamental concepts in geometry that are closely connected.

• Interior Angles
  Interior angles are the angles formed by the intersection of two adjacent sides within a polygon.
  
• Exterior Angles
  Exterior angles are the angles formed by the intersection of a side of the polygon and the extension of its adjacent side.
  

A Practical Example

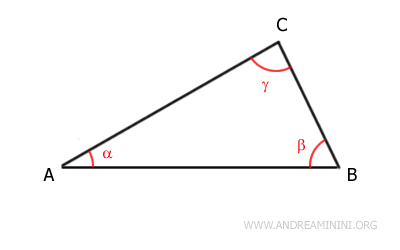
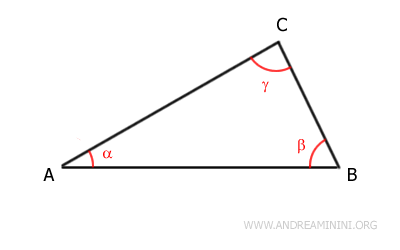
Consider triangle ABC.

The triangle has three interior angles: α, β, and γ.

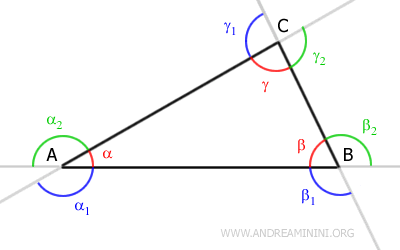
Each interior angle has two adjacent exterior angles.

For example, angle α has two exterior angles: α₁ and α₂.

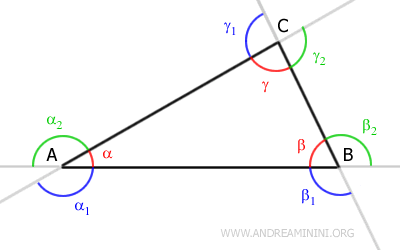
The sum of an interior angle and one of its adjacent exterior angles is always 180° (a straight angle).

Key Observations

Here are some important points about the sums of angles:

• Sum of Interior Angles
  The sum of the interior angles of a polygon with n sides is given by the formula: $$ (n-2) \cdot 180° $$

  Example. A triangle has n=3 sides and 3 interior angles. Their sum is always 180° because $$ (n-2) \cdot 180° = (3-2) \cdot 180° = 1 \cdot 180° = 180° $$

• Sum of Exterior Angles
  The sum of the exterior angles is always 360° regardless of the number of sides of the polygon.

  Example. A triangle has n=3 sides and 3 interior angles. Each side has two exterior angles. However, at any given time, only 3 of these exterior angles can be considered (one for each vertex) and their sum is always 360°.

• Relationship Between Interior and Exterior Angles
  In a polygon, for each angle, the sum of the interior angle and its adjacent exterior angle is always 180°, forming a straight angle.

And so on.