Circumference Length

You can calculate the circumference of a circle with the formula: $$ C = 2\pi r $$

In this formula, \(C\) is the circumference, \(\pi\) (Pi) is a constant approximately equal to 3.14159, and \(r\) is the radius, or the distance from the center of the circle to any point along its edge.

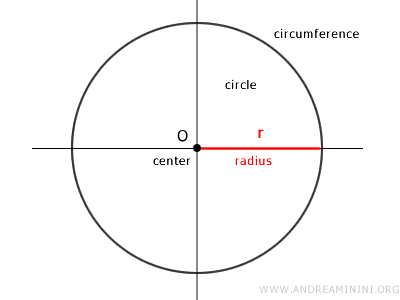
Considering the diameter \(d = 2r\) is twice the radius, you can also express the circumference as:

$$ C = \pi d $$

This equation highlights that the ratio of a circle's circumference (C) to its diameter (d) is a constant value, known as "Pi", independent of the circle's size.

$$ \pi = \frac{C}{d} = 3.1415... $$

The discovery of Pi is deeply rooted in history, with the ancient Egyptians and Babylonians among the first to hint at its existence.

Yet, it was the Greeks who first calculated its value with significant accuracy.

In essence, no matter the size of the circle you draw, the ratio of its circumference to its diameter will always equal approximately 3.1415... Pi is an irrational number with an endless sequence of digits, typically rounded off to 3.14 for simplicity.

And so forth.