Radius of an Inscribed Circle in a Triangle
The radius of a circle inscribed within a triangle is determined by dividing the triangle's area (A) by its semiperimeter (p). $$ r = \frac{A}{p} $$
This equation highlights a captivating link between the triangle's area, its perimeter, and the radius of the encircled inscribed circle.
It's a useful tool for tackling problems related to the characteristics of triangles and circles.
A Practical Example
Consider triangle ABC as our subject.
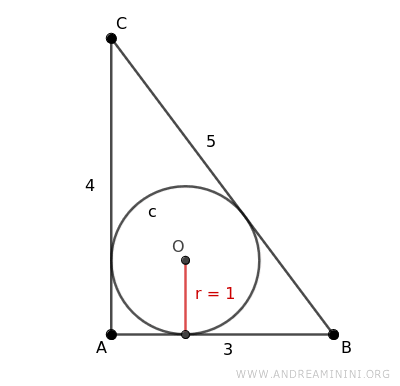
With the sides of the triangle measuring AB=3, AC=4, BC=5, and the radius of the inscribed circle being r=1.
Thus, the semiperimeter of triangle ABC is p=6.
$$ 2p = 3+4+5 $$
$$ 2p = 12 $$
$$ p = 6 $$
Calculating the triangle's area as A=6, I use the formula involving half the product of the base AB=3 and the height AC=4.
$$ Area_{ABC} = \frac{ \overline{AB} \cdot \overline{AC} }{2} $$
$$ Area_{ABC} = \frac{ 3 \cdot 4 }{2} = \frac{12}{2} = 6 $$
Now, let's find the ratio of the area A=6 to the semiperimeter p=6 of the triangle.
$$ r= \frac{Area_{ABC}}{p} = \frac{6}{6}=1 $$
This calculation reveals the length of the radius for the inscribed circle.
Note: For simplification, this example assumes a right triangle, but the principle is applicable across all triangle types.
The Proof
Consider any triangle, labeled ABC.
To locate the incenter—the heart of the inscribed circle—I draw the angle bisectors of triangle ABC.

This inscribed circle touches each side of the triangle at points D, F, and G. As a result, segments EF, EG, and EH are the radii of the circle, all equal by definition: EF≅EG≅EH.
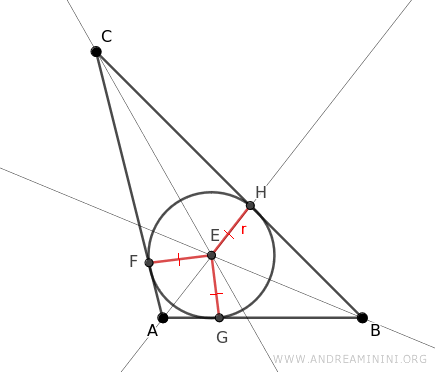
Moreover, these radii are perpendicular to the triangle’s sides where they touch, marking them as the heights of the sub-triangles ACE, ABE, and BCE within ABC.
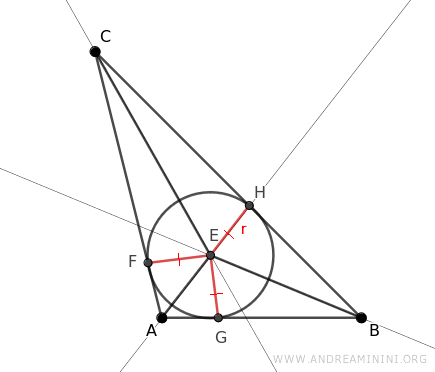
The areas of sub-triangles ACE, ABE, and BCE collectively equal the area of the larger triangle ABC, showcasing a beautiful symmetry. Thus, the aggregate of the areas of ACE, ABE, and BCE matches the area of ABC.
$$ \text{Area}_{ABC} = \text{Area}_{ACE} + \text{Area}_{ABE} + \text{Area}_{BCE} $$
Calculating the area of a triangle involves halving the product of its base and height. Here, for sub-triangles ACE, ABE, and BCE, the base is a side of ABC, and the height is the radius (r) of our inscribed circle.
$$ \text{Area}_{ABC} = \frac{ \overline{AC} \cdot r }{2} + \frac{ \overline{AB} \cdot r }{2} + \frac{ \overline{BC} \cdot r }{2} $$
Isolating the radius r:
$$ \text{Area}_{ABC} = r \cdot \left( \frac{ \overline{AC} }{2} + \frac{ \overline{AB} }{2} + \frac{ \overline{BC} }{2} \right) $$
$$ \text{Area}_{ABC} = r \cdot \left( \frac{ \overline{AC} + \overline{AB} + \overline{BC} }{2} \right) $$
The sum of the sides AC+AB+BC=P forms the perimeter (P) of triangle ABC, equivalently double its semiperimeter P=2p.
$$ \text{Area}_{ABC} = r \cdot \left( \frac{ 2p }{2} \right) $$
$$ \require{cancel} \text{Area}_{ABC} = r \cdot \left( \frac{ \cancel{2} p }{ \cancel{2} } \right) $$
$$ \text{Area}_{ABC} = r \cdot p $$
This elegantly proves that the area of triangle ABC equals the product of the inscribed circle's radius (r) and the triangle's semiperimeter (p).
And that's just the beginning.
