Pythagoras' theorem
The Pythagorean Theorem is applicable to right triangles, which are triangles featuring a 90-degree angle. Its principle is articulated as follows:
Within a right triangle, the area of the square constructed on the hypotenuse (the side opposite the right angle) equals the sum of the areas of the squares built on the other two sides. $$ a^2 + b^2 = c^2 $$ Here, "c" represents the hypotenuse, while "a" and "b" denote the legs of the triangle.
This theorem bears the name of the ancient Greek mathematician Pythagoras, who is traditionally credited with its first formulation.
Its Purpose
The theorem provides a method for calculating the lengths of the sides in a right triangle.
For example, by knowing the measurements of two sides, it allows the determination of the length of the third side.
Theorem Applications. The Pythagorean Theorem finds its use across a broad spectrum of applications in mathematics, geometry, and physics. It's instrumental in computing distances between points in a space and forms the foundation for the definitions of the trigonometric functions: sine, cosine, and tangent.
Even though this theorem was established millennia ago, it remains a cornerstone of modern science, contributing to numerous other geometric, mathematical, and physical proofs and theories.
A Hands-on Example
Let's consider a right triangle.
The two legs adjacent to the right angle are 4 and 3 units in length.
$$ a=4 \\ b=3 $$
Yet, the length of the hypotenuse (side c), the side opposite the right angle, is unknown.
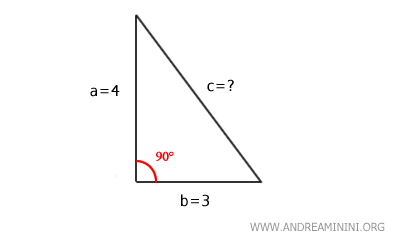
Using the measurements of the adjacent sides, the Pythagorean Theorem enables the calculation of the hypotenuse.
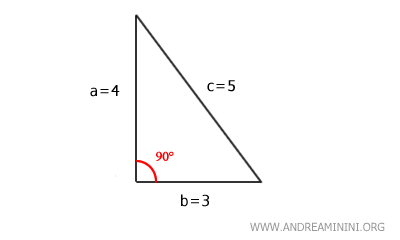
The square of the hypotenuse (c2) is equal to the sum of the squares on the legs (a2+b2).
$$ c^2 = a^2 + b^2 $$
With the known side lengths a=4 and b=3, we substitute these values.
$$ c^2 = 4^2 + 3^2 $$
$$ c^2 = 16 + 9 $$
$$ c^2 = 25 $$
Applying the equation's invariance property, I proceed to extract the square root of both sides.
$$ \sqrt{c^2} = \sqrt{25} $$
$$ c = 5 $$
Therefore, the length of the hypotenuse is determined to be c=5.
Likewise, knowing one leg and the hypotenuse enables the calculation of the other leg's length. $$ a^2 = c^2 - b^2 $$ $$ b^2 = c^2 - a^2 $$ Keeping the theorem's main formula in mind, c2=a2+b2, simply rearrange to isolate the unknown side on one side of the equation and the known sides on the other.
Proofs
The Pythagorean theorem has a rich history of proofs, some of which span back several millennia.
One particularly elegant and well-known proof adopts a geometric strategy.
Consider a right triangle.

Imagine drawing three squares, each based on the lengths of the triangle's sides.
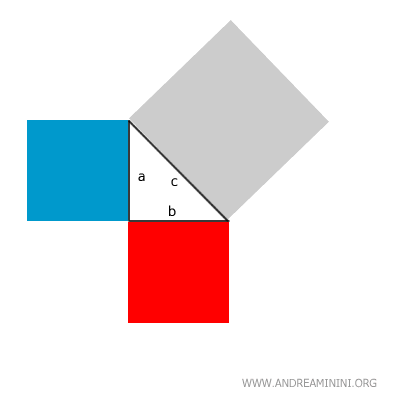
The largest square (in gray) corresponds to the hypotenuse—the triangle's longest side. The other two squares (in red and blue) relate to the shorter sides, or the legs.
Each of the smaller squares is then divided into two right triangles.
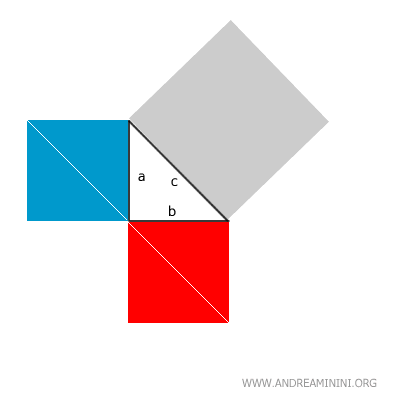
Next, rearrange these pieces to exactly fill the area of the largest square.
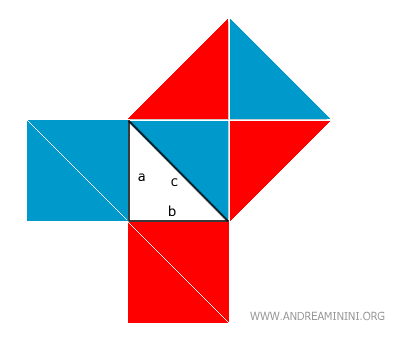
This arrangement visually proves that the area of the largest square is precisely the sum of the two smaller squares' areas, thus affirming the theorem's truth.
An Alternate Proof
A different approach to proving the Pythagorean theorem involves Euclid's principle.
First, squares are constructed on each of the triangle's sides, including the hypotenuse.

The height (h) of the triangle is drawn with the hypotenuse (c) as its base, effectively projecting the legs onto the hypotenuse via a perpendicular.
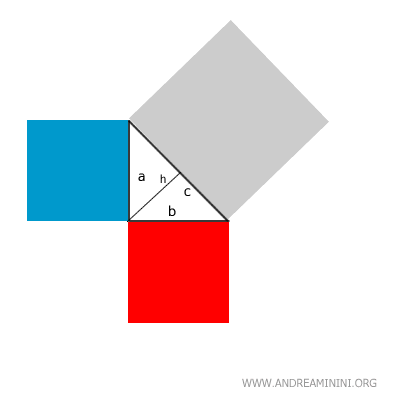
This height extends, bisecting the square on the hypotenuse.
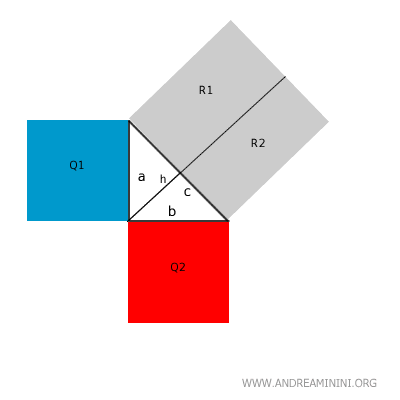
The hypotenuse square's area then becomes the sum of areas R1 and R2.
By Euclid's first theorem, the area of the rectangle formed by projecting leg "a" onto the hypotenuse matches the area of the square on leg "a", denoted as Q1=R1.
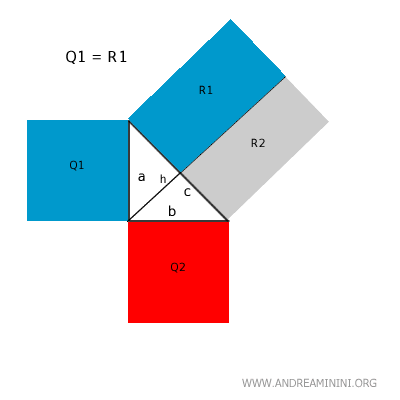
Similarly, the rectangle formed by projecting leg "b" aligns in area with the square on leg "b", denoted as Q2=R2, again following Euclid's first theorem.

Concluding that the hypotenuse square's area equals the sum of the two rectangles (R1+R2) and thus the sum of the squares on the legs (Q1+Q2), solidifies the theorem.
$$ R1+R2 \doteq Q1+Q2 $$
This elegantly confirms the Pythagorean theorem.
Note: This is but a glimpse into the myriad of proofs validating the Pythagorean theorem, showcasing just two of the numerous demonstrations available.
Insights
Here are some further insights and notes about the Pythagorean theorem.
- The Converse of the Pythagorean Theorem
In any triangle, if the sum of the squares of two sides is equal to (shares the same area as) the square on the third side, then that triangle is a right triangle.
- The Generalized Pythagorean Theorem
In a right triangle, when you construct three similar polygons on each of the triangle's sides, the area of the polygon on the hypotenuse (A3) equals the combined areas (A1+A2) of the polygons on the other two sides.

- The Origins of the Pythagorean Theorem
According to legend, Pythagoras came upon his famous theorem while waiting for an audience with the tyrant Polycrates in Samos. Observing the square tiles of the waiting room floor, he noticed one tile was cracked diagonally, creating two isosceles triangles. Imagining a square constructed along the diagonal of the halved tile, Pythagoras realized its area matched exactly the sum of the areas of squares built on the two remaining sides of the right triangle. This tale beautifully illustrates how profound insights can stem from the simplest of everyday occurrences—a cracked tile or an apple falling from a tree. - Pythagorean Triple
A Pythagorean triple is a set of three positive integers (a, b, c) where the sum of the squares of the first two numbers equals the square of the third number: $$ a^2 + b^2 = c^2 $$
And so forth.
