Semicircle

A semicircle is essentially half of a circle.

Drawing a diameter across a circle effectively divides it into two equal halves, each being a semicircle.

The circumference of a semicircle is simply half that of the full circle.

$$ S_c = \pi \cdot r $$

Explanation: Considering r as the radius of the circle and C=2πr as the full circumference, the length of the semicircle is computed as πr. In formula terms, $$ S_c = \frac{C}{2} = \frac{2 \pi \cdot r}{2} = \pi \cdot r $$

The space between the diameter and the semicircle's curve is referred to as a semicircular region.

The area of this semicircular region is exactly half the area of the entire circle.

$$ A_{sc} = \frac{r^2 \pi}{2} $$

Explanation: Given the circle's area is A=πr^2, the area of the semicircular region follows as $$ A_{sc} = \frac{A}{2} = \frac{r^2 \pi}{2} $$

Insights

Here are some insights and interesting facts about semicircles:

• An angle inscribed in a semicircle is always a right angle.
  This geometric property is frequently leveraged in Euclidean geometry.

  Proof: Imagine a circle centered at O with a diameter AB.
  
  Select any point C on the semicircle that is bounded by A and B. The goal is to prove that angle ACB forms a right angle (90°).
  
  Draw the line OC.
  
  Since OA and OB are radii of the circle and thus equal in length, and OC is also a radius, it too shares this equal length. $$ \overline{OA} = \overline{OB} = \overline{OC} $$ The central angle AOC covers the arc AC and the angle BOC the arc BC. The angle AOB which spans the full arc ACB is 180°, indicative of a semicircle,
  
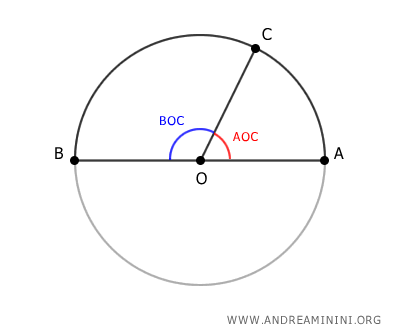
  According to the Inscribed Angle Theorem, an angle inscribed in a circle is half the central angle that spans the same arc. Hence, if the central angle over the arc ACB is a straight angle AOB = 180°, then the inscribed angle ACB is 180°/2, namely 90°, confirming ACB is a right angle.
  

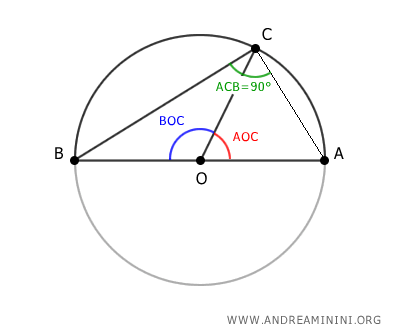
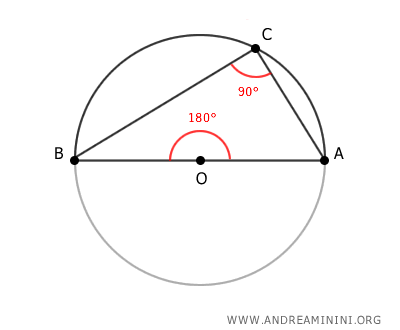
• Any triangle inscribed in a semicircle is a right-angled triangle with the diameter serving as its hypotenuse.
  This inference is also drawn from the Inscribed Angle Theorem. If the central angle equals 180°, then the angle at the perimeter is halved to 90°.
  

And so forth.