Connectivity in Topology

In topology, a space is connected if it is not composed of disjoint open sets. This means that connectivity refers to a space's ability to allow any two points within it to be joined by a path without leaving the confines of the space.

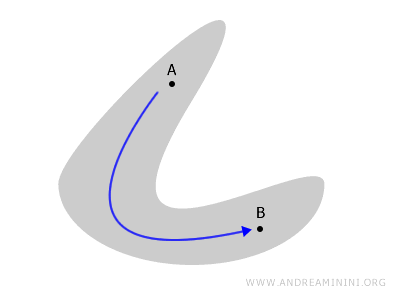
Connectivity describes how parts of a topological space are either linked together or separated.

This concept is fundamental in topology, along with continuity.

Connectivity is crucial in many areas of mathematics as it provides insights into the structure of spaces and the relationships between their various components. For example, it helps in classifying and analyzing topological spaces.

A Practical Example

A space, be it a plane figure or a polyhedron, is considered a connected space if there is a continuous path linking any two points A and B within it, without exiting the space.

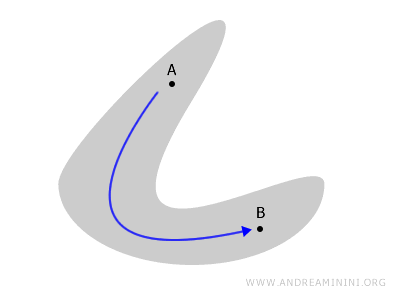
On the other hand, if parts of the space are separated, then the space is not connected (termed a disconnected space).

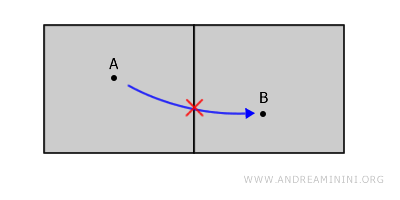
For instance, in this scenario, the space is divided into two disjoint parts, and any path connecting points A and B would exit the space.

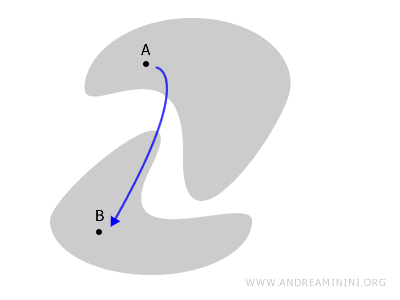
It’s worthwhile to delve deeper into the concept of disconnected spaces with another practical example.

When is a space disconnected?

The simplest illustration of a disconnected space is imagining two separate rooms within the same building, divided by a wall. These rooms represent two open sets that do not include their boundaries (the walls) and are separated.

Although the rooms appear connected, they are not, as any path from point A to B would necessitate passing through the wall, which is outside the defined space.

In summary, it’s essential to remember that boundaries do not constitute part of an open set.

Local Connectivity

Local connectivity occurs when every open set in the space, even if disjoint from others, has a connected neighborhood. This means each point is part of a connected open subset.

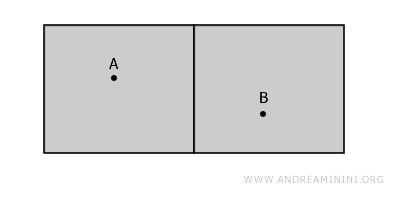
Take, for example, a space made up of disjoint open sets, like the two separate rooms within a building.

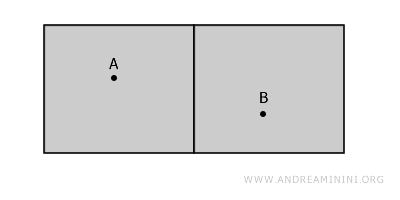
The space is disconnected because there is no way to connect points A and B without crossing the walls.

However, at point A, there exists a subset that encapsulates it and where all points are interconnected, demonstrating local connectivity.

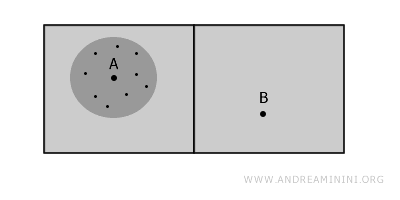
Similarly, point B also exhibits local connectivity.

Types of Connections

While there are many forms of connectivity, the two most prevalent are:

• Path Connectivity
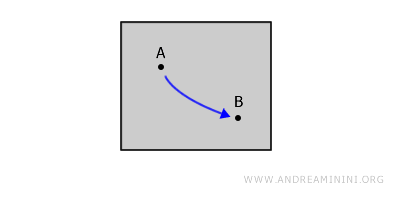
  A topological space is path-connected if any two points, A and B, can be linked by a continuous path, a journey that remains within the space. This type of connectivity suggests that all path-connected spaces are inherently connected, although the reverse may not always hold true.
  

  For instance, consider a sealed planar shape. Any two points within can be joined by a continuous path that never breaks the plane’s surface.
  

• Simple Connectivity
  A space is simply connected if any closed loop within it can be contracted to a point. This indicates that the space is unified without any internal voids. A simply connected space is always connected, but not all connected spaces are simply connected. In formal terms, in a simply connected topological space, every loop is homotopic to a point.
  

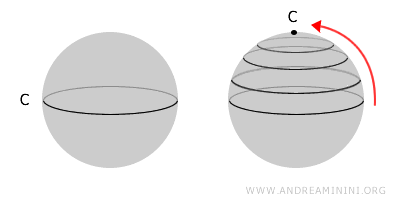
  A sphere, for example, is simply connected because any loop on its surface can be shrunk to a point. Conversely, a doughnut, with its intrinsic hole, cannot reduce every loop to a point, highlighting its connected but not simply connected nature.
  
  
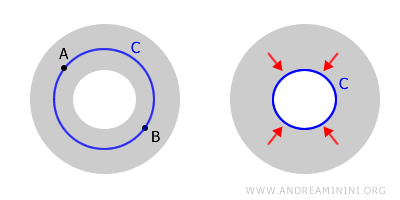
  Such a space, connected yet not simply connected, is termed multiply connected. An annular space is a prime example of this type of connectivity.

Observations

Here are a few personal notes and observations:

• In the realm of real numbers, the only connected spaces are intervals.