First Triangle Congruence Theorem

The first triangle congruence theorem states that two triangles are congruent if they have two sides and the included angle congruent in the same order.

In other words, two triangles are congruent if two sides and the included angle are identical in both triangles.

This congruence theorem is also known as the SAS (Side-Angle-Side) criterion.

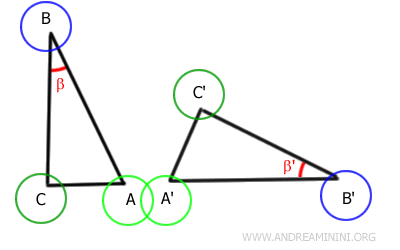
For example, consider these two triangles ABC and A'B'C'

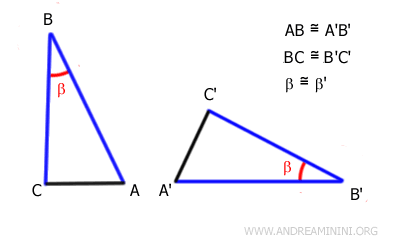
The sides AB and BC of the first triangle are respectively congruent to the sides A'B' and B'C' of the second triangle, meaning they have the same length even though their orientations are different.

$$ \overline{AB} \cong \overline{A'B'} $$

$$ \overline{BC} \cong \overline{B'C'} $$

Additionally, the angles β and β' between these pairs of sides are congruent, meaning they have the same measure.

$$ \beta \cong \beta' $$

Therefore, according to the first congruence theorem, the two triangles are congruent.

If the congruent angle is not included between the two sides, the triangles are not necessarily congruent. For example, consider triangle ABC. Then, draw a circle with radius AC and center A. The arc intersects the extension of segment AC at point D. In this way, you can draw another triangle ACD with two sides congruent to the previous triangle.

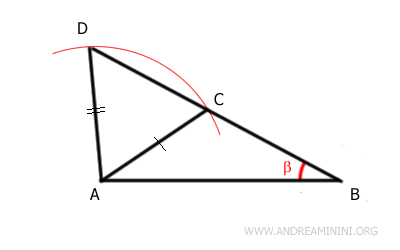
It is evident that the two triangles ABC and ABD are not congruent because they are not superimposable. Triangle ABD is larger than triangle ABC. However, both triangles have two congruent sides, AB ≅ AB and AC ≅ AD, and a congruent angle β. But the angle β is not included between the two congruent sides. In conclusion, two congruent sides and a non-included angle are not sufficient to declare the congruence of the triangles.

Proof

The first congruence theorem is considered a postulate because it is based on Euclid's axioms.

Therefore, what follows is not a formal proof, as rigid motions are only intuitive concepts.

Consider two triangles ABC and A'B'C'

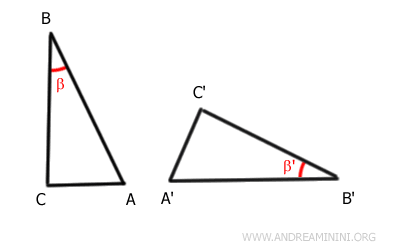
With a rigid motion, side AB overlaps side A'B'

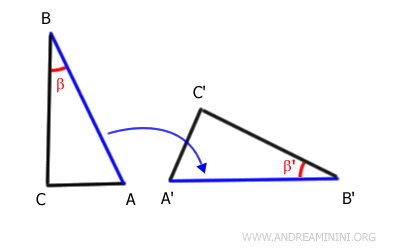
Therefore, the endpoints overlap. Point A overlaps point A' and point B overlaps point B'

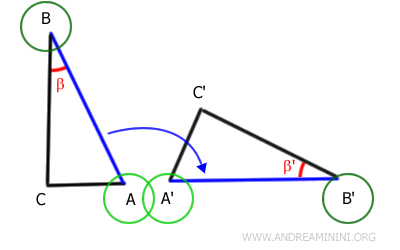
The angles β and β', adjacent to sides AB and A'B', are also overlapping, so they have the same measure.

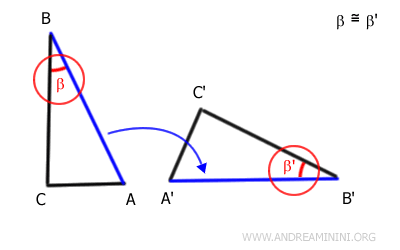
Consequently, the rays containing sides BC and B'C' also overlap.

The sides BC and B'C' are also overlapping.

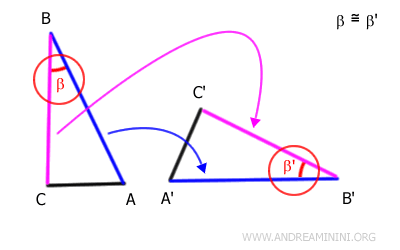
This means that the endpoints of the two sides also overlap. Point B overlaps point B' (as already known) and point C overlaps point C'.

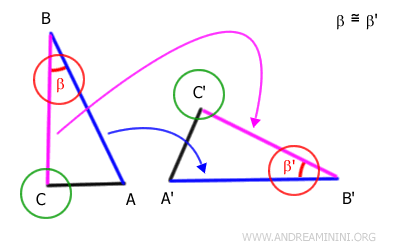
In conclusion, all the vertices of the two triangles overlap after the rigid motion.

Therefore, all the sides and angles of the two triangles are congruent in an orderly manner.

This proves that the two triangles are congruent.

And so on.