Postulates

Postulates (or axioms) are properties accepted as true without proof, serving as the basis for deriving other truths logically.

The term "postulate" originates from the Latin "postulatum," which means "that which is assumed."

An Example of a Postulate

Here is an example of a postulate or axiom:

According to Euclid's first postulate, "A straight line can be drawn from any one point to any other point."

This postulate states that if you have two distinct points, A and B, you can always draw a straight line connecting them.

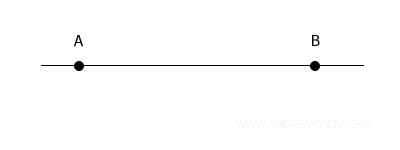
Euclid's first postulate is a simple proposition that doesn't require proof or derivation and is accepted as true.

However, it is also a fundamental starting point for many proofs and geometric constructions in Euclidean geometry.

The Difference Between Postulates and Axioms

Originally, in Greek philosophy, the terms "postulate" and "axiom" had slightly different meanings:

• A postulate was a proposition accepted as true without proof because it served as a starting point for reasoning or argument. For example, Euclid's postulates in geometry.

  Note: For the Stoics, postulates were simply statements that could be true or false; they were not necessarily undeniable truths.

• An axiom was considered a fundamental or self-evident truth that did not require proof. Axioms were seen as universal principles true by their very nature.

  Example: Aristotle's "Principle of Non-Contradiction" states that a proposition and its negation cannot both be true at the same time. In other words, if a statement is true, then its opposite must be false, and vice versa. For instance, if the statement "It is day" is true, then the statement "It is not day" must be false. This principle is the basis of classical logic and is considered an axiom because it is a fundamental truth that does not require proof.

Over time, the meanings of axiom and postulate have evolved, and the distinction between them has blurred.

Until the 18th century, axioms were considered "self-evident" truths accepted without proof.

With the advent of non-Euclidean geometries, the notion of self-evidence was discarded. From the late 19th century onwards, the terms "postulate" and "axiom" have been used interchangeably.

Today, axioms and postulates are statements that, within a logical system, are not deducible from other propositions but serve as the foundation from which other propositions, theories, or theorems are derived.

They are premises accepted by convention or for operational convenience, provided they do not generate contradictions, and they need not be self-evident.

And so on.